Question

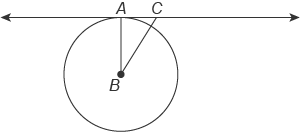
AC←→ is tangent to the circle with center at B. The measure of ∠ACB is 58°. What is the measure of ∠ABC ?

Answer 1

`angleABC=32^@`

Explanation:

`angleBAC=90^@to(" angle between tangent and radius")`

`"angle sum of "triangleABC=180^@`

`rArrangleABC=180^@-(90+58)^@=32^@`

Answer 2

58°

Explanation:

The answer is given in the question

Answer 3

`angleABC = 32^@`

Explanation:

Let's start out by laying out what we know:

1) `stackrel_"AC"` is tangent to the circle

2) Since `stackrel_"AC"` is tangent to the circle, we know that it forms a right angle with the radius `stackrel_"AB"`. So `angleA = "right angle" = 90^@`

3) `/_ACB=58^@` (this angle is the same as `angleC` which is what I'm going to call it.)

Do you see that little triangle that's inside the circle? Well we know that the angles of a triangle add up to `180^@`, we can make this into an equation:

`/_A + /_ABC + /_C = 180^@`

Let's substitute into this equation since we know some of the angles:

`angleA + angleABC + angleC = 180^@`
`90^@ + angleABC + 58^@ = 180^@`
`148^@+ angleABC = 180^@`
`angleABC = 32^@`