How do you graph #y=2x^2+12x+16#?
1 Answer
Mar 19, 2018
There is a procedure to graph funcion.
Explanation:
- Define the demain and codomain: polynoms are defined everywhere in the real plane (complex too).
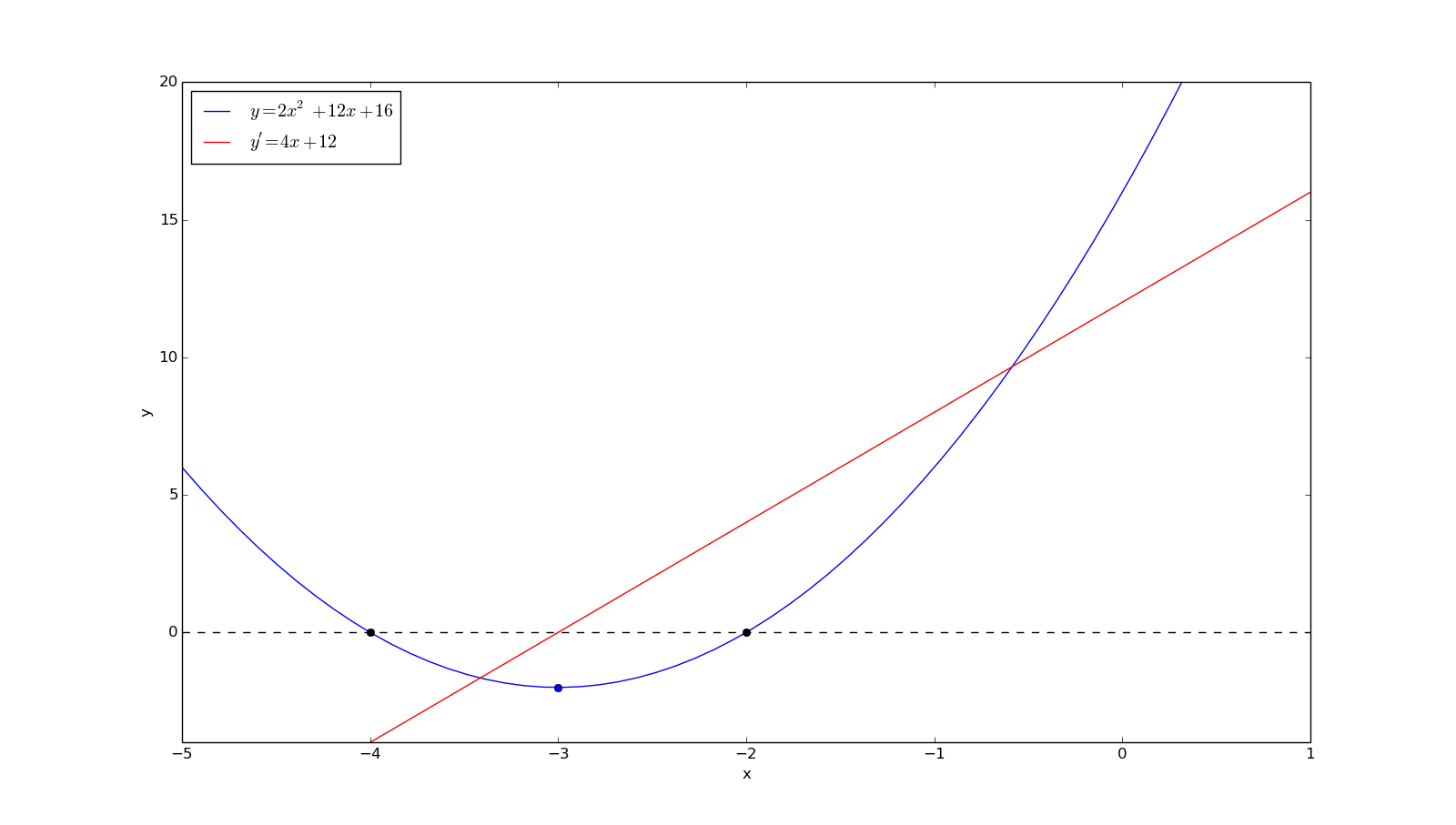
#y=2*x^2+12*x+16# - Find the intersection between function and x-axes: solve the equation
#2*x^2+12*x+16=0# | :2
#x^2+6*x+8=0#
Remember:#(a+x)*(b+x)=x^2+(a+b)*x+a*b#
The solution is:#x^2+6*x+8=(x-4)*(x-2)=0# #x_1=4, x_2=2#
[black points] - Calculate the first derivative:
#y'=4*x+12# - Calculate
#y'=0# :
#4*x+12=0# ,#x=-3#
here#(-3,y(-3))=(-3,-2)# blue point the slope change.#(-infty,-3)# the slope is negative (the value of the function fall),#(-3,+infty)# the slope is positive (the value of the function increase). - Calculate the second derivative:
#y''=4#
if#y''>0 # the function is convex (is smiling)
if#y''<0 # the function is concave (is sad)
#y''=4 >0 rArr# is convex
( )
)