Question

How do you simplify `8( m - 5) + 6m`?

Answer 1

`14m-40`

Explanation:

you use the distributive property on `8(m-5)` to get `8m-40`. you put that into the equation and get `8m-40+6m`. then you combine like terms

Answer 2

First expand, then simplify, and you will get
`y=14m-40`

Explanation:

Expand:

`underline(8(m-5))+6m`

`=8m-40+6m`

Now simplify:

`=underline8m-40+underline6m`

`=14m-40`

Answer 3

`8(m-5)+6m`
`8m-40+6m`
`8m+6m-40`
`14m-40`

Answer 4

`14m - 40`

Explanation:

We will use the distributive property to solve this equation. Here is a helpful picture about the property:

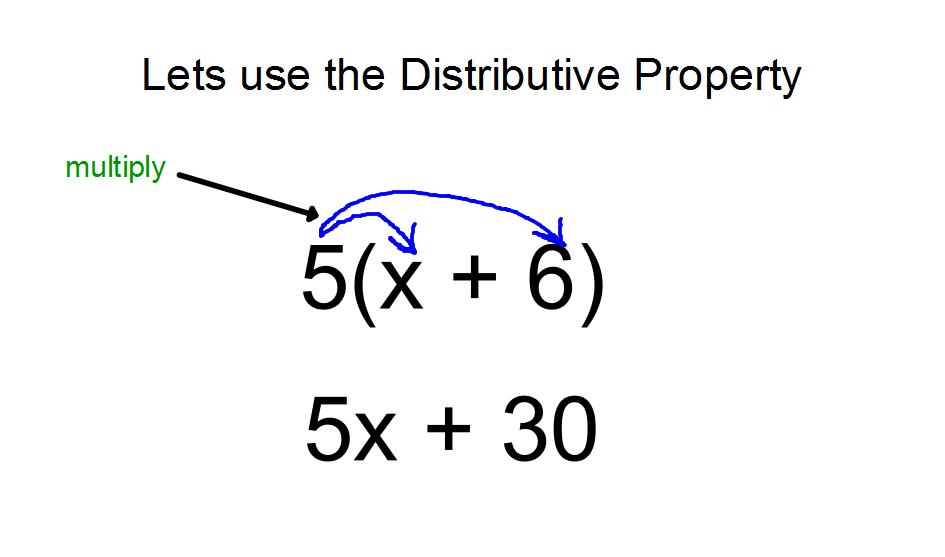
So we are going to multiply `8` by `m` and `8` by `-5`

`8(m-5) + 6m`

`8(m) + 8(-5) + 6m`

`8m + (-40) + 6m`

`8m - 40 + 6m`

`14m - 40`

Hint: when you see the distributive property in a problem, it's easiest to draw arrow like it shows in the picture, so that you know what to multiply together. It's really quick, and it will help you get the problems right!

Answer 5

`14m-40`

Explanation:

1st you distribute `(8*m)` and `(8*-5)` Because when you have a number. Next you parentheses you multiply it by everything in the parentheses (in most cases). So then you have `8m-40+6m` and you add `8m` and `5m` together because are common factors. Then you get `14m-40`. Bada Bing Bada Boom.