Math Help???! Please?
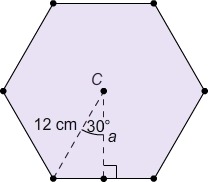
The base of a regular pyramid is a hexagon.
What is the area of the base of the pyramid?
Express your answer in radical form.

The base of a regular pyramid is a hexagon.
What is the area of the base of the pyramid?
Express your answer in radical form.
2 Answers
Explanation:
A regular hexagon can be divided into six congruent triangles,
area of
Area of the regular hexagon
Side notes : formula for a regular hexagon is
given that
Where, c: distance from center to hexagon corner = 12
Explanation:
Finding the rectangle high "a" from hexagon center to center of one hexagon side "c/2"
Or,
The hexagon area will be 6 times the equilateral triangle:
Finally,
Where, c: distance from center to hexagon corner
If c = 12
Then

