Help please?
In the cast diagram how do w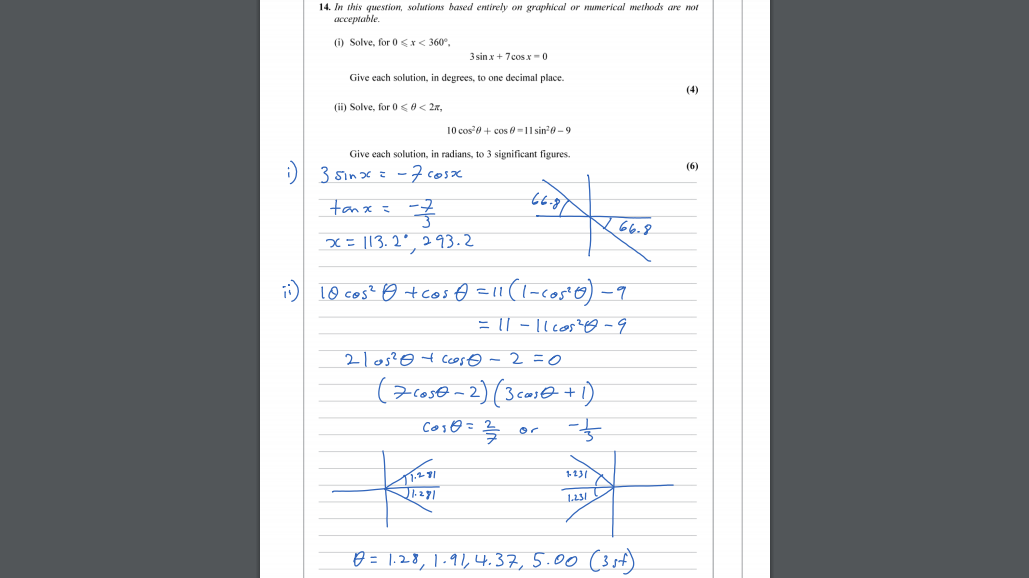 e know whether we're supposed to go anticlockwise or clockwise? In part a) tanx is negative so WHY are we going anticlockwise (ie 180 - 66.8 and 360 - 66.8)? And in part b I dont get the cast diag. Could someone pls help me out with this?
e know whether we're supposed to go anticlockwise or clockwise? In part a) tanx is negative so WHY are we going anticlockwise (ie 180 - 66.8 and 360 - 66.8)? And in part b I dont get the cast diag. Could someone pls help me out with this?
In the cast diagram how do w e know whether we're supposed to go anticlockwise or clockwise? In part a) tanx is negative so WHY are we going anticlockwise (ie 180 - 66.8 and 360 - 66.8)? And in part b I dont get the cast diag. Could someone pls help me out with this?
e know whether we're supposed to go anticlockwise or clockwise? In part a) tanx is negative so WHY are we going anticlockwise (ie 180 - 66.8 and 360 - 66.8)? And in part b I dont get the cast diag. Could someone pls help me out with this?
2 Answers
For the first part:
tan will give the negative value at the 1st and fourth quadrant only
For question 2:
On the right hand side, you need to apply a trigonometry identity which is
Then rearrange the equation so that all will be on the left hand side, which will give:
Let
therefore
Factor the equation:
Substitute back
Then notice that theta give positive value on 1st and 4th quadrant while negative value on 2nd and 3rd quadrant
I hope that helps!
Please see below.
Explanation:
.
Whenever you solve for an angle from a trigonometric equation, you always have to go counterclockwise to get a positive angle and clockwise to get the a negative angle that has the same magnitude.
Again, based on the explanation in
If after reaching
Similarly, if after reaching
All four angles are in quadrants
Similarly:
Following the same process as before, we go


