How to solve this question?

Screenshot

4 Answers
Tom M.
Apr 15, 2018

a) Not answer
b) not answered
c) #L/Msqrt(k(M+m)#
d)#2L#
Read disclaimer

Explanation:

Disclaimer: I'm fairly sure my method is not that expected from the question, but I found it interesting and wanted to share my attempt anyway. I think its wrong because I effectively skipped parts a and b. Any feedback on this would be great!

Assumptions I've made which might be wrong:

  • all the energy transferred in the spring is transferred into kinetic energy for the marble and piston when the piston returns to equilibrium
  • the marble and piston travel together as a single particle until the piston returns to equilibrium piston, then the marble is projected off.

Consider the problem in stages.

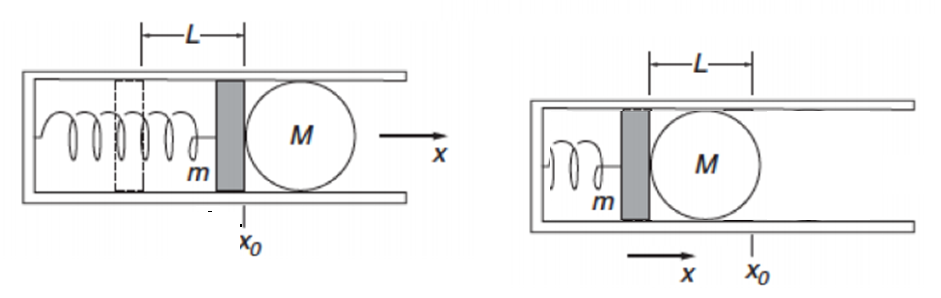
enter image source here

#"Energy in stretching the string"=1/2kL^2#

Due to the conservation of energy, this will all be transformed to kinetic energy when the piston returns to #x_0#, its starting position.

This is the kinetic energy possessed by the whole system, this being the piston and marble.

Let #x# be the final speed of the marble and piston combined.

#E_k=1/2(M+m)x^2#

#1/2kL^2=1/2(M+m)x^2#

#x^2=(kL^2)/(M+m)#

#color(blue)(x=sqrt((kL^2)/(M+m)#

Now, consider when the marble slips from the piston.

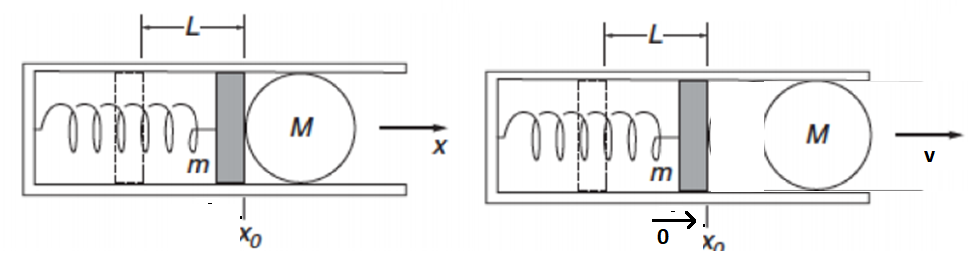
enter image source here

The piston will be stationary at the end, so has a final velocity of 0.
We can find the final speed of the marble using the conservation of momentum.

From conservation of momentum
Total momentum before = total momentum after

#(M+m)sqrt((kL^2)/(M+m))=Mv#

#Mv=sqrt((M+m)^2)sqrt((kL^2)/(M+m))#

#=sqrt((kL^2(M+m)^cancel2)/cancel(M+m))#

#M^2v^2=kL^2(M+m)#

#v^2=L^2/M^2k(M+m)#

#color(blue)(v=L/Msqrt(k(M+m))#

for part d), we will do the inverse.

Let the marble be projected off at speed #2v#

enter image source here

From conservation of momentum
Momentum before=momentum after

#2Mv=x(M+m)#

#2Lsqrt(k(M+m))=x(M+m)#

#color(blue)(x=(2Lsqrt(k(M+m)))/(M+m)#

Now, we'll use our energy idea from earlier to find our new length back for the spring, #L_1#

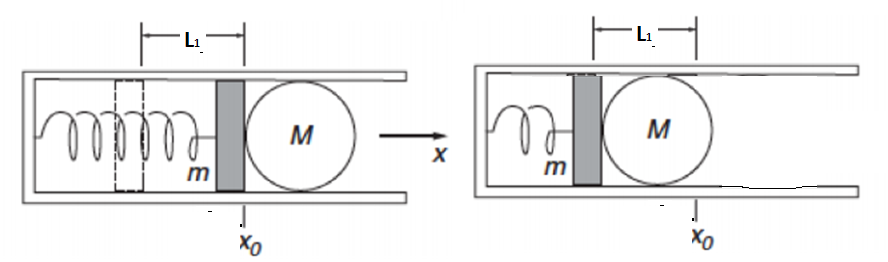
enter image source here

#KE=1/2(M+m)((2Lsqrt(k(M+m)))/(M+m))^2#

#=cancel(M+m)/2*(4L^2kcancel((M+m)))/cancel((M+m)^2)#

#=2kL^2#

#E_"pot" " in spring"=1/2kL_1^2#

By conservation of energy;

#1/2cancelkL_1^2=2cancelkL^2#

#L_1^2=4L^2#

#color(blue)(L_1=2L#

So to get double the speed out, double the distance you pull the spring. (rather boring final answer, if I do say so).

Cesareo R.
Apr 16, 2018

See below.

Explanation:

Movement equation

#k(x-x_0) + (m+M)d^2/(dt^2)(x-x_0) = 0#

Multiplying by #d/(dt)(x-x_0)#

#1/2 k d/(dt)(x-x_0)^2+(m+M)1/2 d/(dt)(d/dt(x-x_0))^2 = 0#

Calling #x-x_0 = y# and #d/(dt)(x-x_0) = v# we have after integration

#1/2 y^2+1/2(m+M)v^2 = C#

this relationship works just until the marble loses contact with the piston.

Also integrating directly the movement equation

#k y +(m+M) ddot y = 0#

which is a harmonic movement equation results

#y = y_0 sin(sqrt(k/(m+M))t+phi_0)#

and

#v = dot y = sqrt(k/(m+M))y_0 cos(sqrt(k/(m+M))t+phi_0)#

A08
Apr 16, 2018

See parts (c) and (d) as a separate answer.

Explanation:

(a) Keeping in view the given figure, and considering #x=x_0# as the origin, equation of motion for the system can be obtained from Newton's Second Law of Motion and Hook's Law as

#F_"net"=(M+m)(d^2x)/dt^2=-kx#

Rewriting it as a second order differential equation of SHM.

#(d^2x)/dt^2+(k)/(M+m)x=0# ......(1)

(b) Above equation has a general sinusoidal solution as

#x=Asin\ omegat+B cos\ omega t# ......(2)
where #omega=sqrt((k)/(M+m))#

Now velocity is given by

#dot x=omegaAcosomegat-omegaBsinomegat# ......(3)

Displacement at #t=0# is #=-L#. From (2) we get

#-L=Asin\ (omegaxx0)+B cos\ (omega xx0)#
#=>B=-L #

Velocity at #t=0# is #=0#. From (3) we get

#0=omegaAcos(omegaxx0)-omegaBsin(omegaxx0)#
#=>A=0#

Solution (2) becomes

#x=-L cos\ omega t#

Velocity becomes

#dot x=omegaLsinomegat#

It is clear that equation of motion and its solution are valid so long as piston and marble move together as single entity.

A08
Apr 16, 2018

From other posted solution we got

Position

#x=-L cos\ omega t# ......#(i)#

Velocity

#dot x=omegaLsinomegat# ........#(ii)#

Explanation:

(c) Let velocity of the piston marble system be maximum at #t=T#. After this point, piston starts to slow down, the contact between the two is lost and marble carries on its forward motion with this constant velocity. Due to presence of #sin omegat# factor in the expression for velocity, maximum velocity is reached when

#sinomegaT=1#
#=>omegaT=pi/2#

#:.# from #(ii)#

#dotx_max=omegaL#
#=>dotx_max=sqrt(k/(m+M))L# ......#(iii)#

Inserting value of #omegaT# in expression for position we get

#x(T)=-L cos\ (pi/2)#
#x(T)=0=x_0#

(d) From #(iii)# we see that

#dot x_maxpropL#

Therefore, in order to achieve twice the speed of marble on separation the piston must be pushed/pulled back twice the initial value.

#=2L#

Related questions
Impact of this question
1956 views around the world
You can reuse this answer
Creative Commons License