In triangle ABC, a = 3, b = 7, and angle C = 86º. Find the area of the triangle correct to the nearest tenth?
1 Answer
Apr 24, 2018
Explanation:
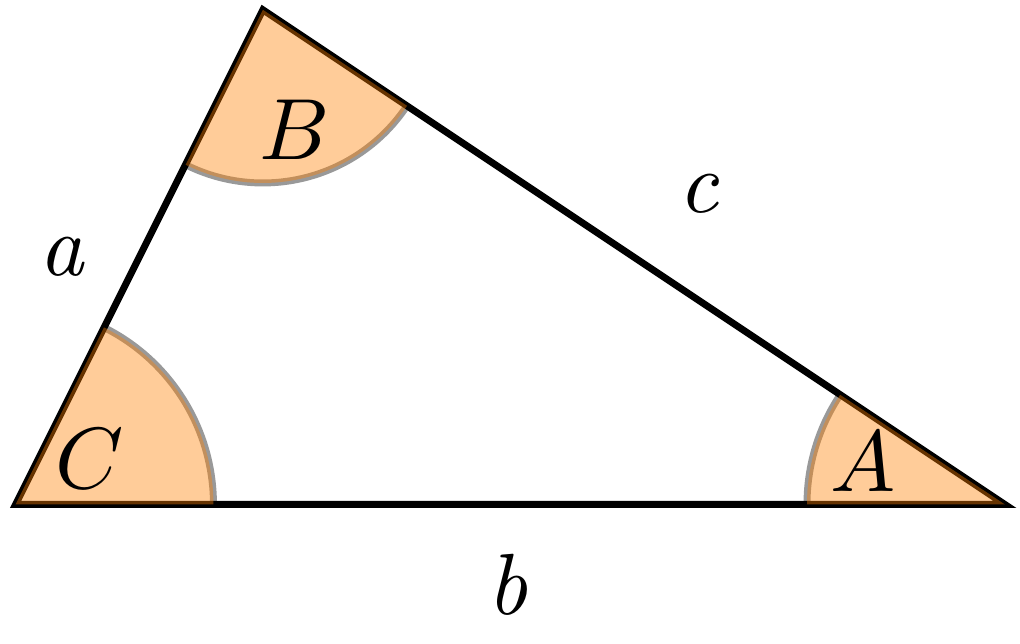
The area of a triangle is:
If we draw a line from the top angle
There are other methods to calculate the area of a triangle, sometime Heron's formula can be useful.