.
#x=y^(2/3)-1#
#y=x^(1/3)+1#
#y^(2/3)=x+1#
#y^2=(x+1)^3#
#y^2=(root3x+1)^2#
#(x+1)^3=(root3x+1)^2#
Let #u=root3x#
#(u^3+1)^3=(u+1)^2#
#u^9+3u^6+3u^3+1=u^2+2u+1#
#u^9+3u^6+3u^3-u^2-2u=0#
#u(u^8+3u^5+3u^2-u-2)=0#
#u=0, :. root3x=0, :. x=0, y=1#
#(0,1)# is one intersection point.
#u^8+3u^5+3u^2-u-2=0#
This equation can be solved by a using rational roots theorem and Newton-Raphson method; and will yield the following results:
#u=-1, 0.77541, -0.95795#
Setting each one equal to #root3x# yields the three #x# values:
#x=-1, 0.46622, and -0.87907#
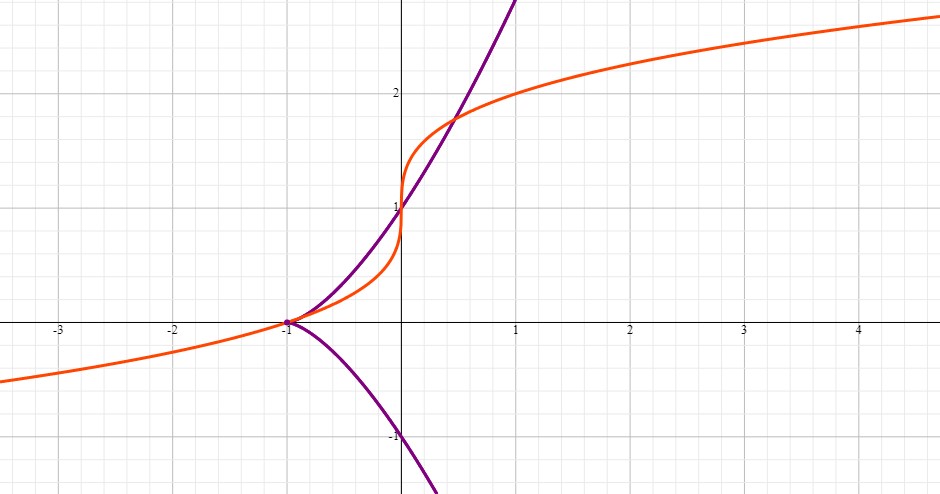
Therefore, we have four points of intersection:
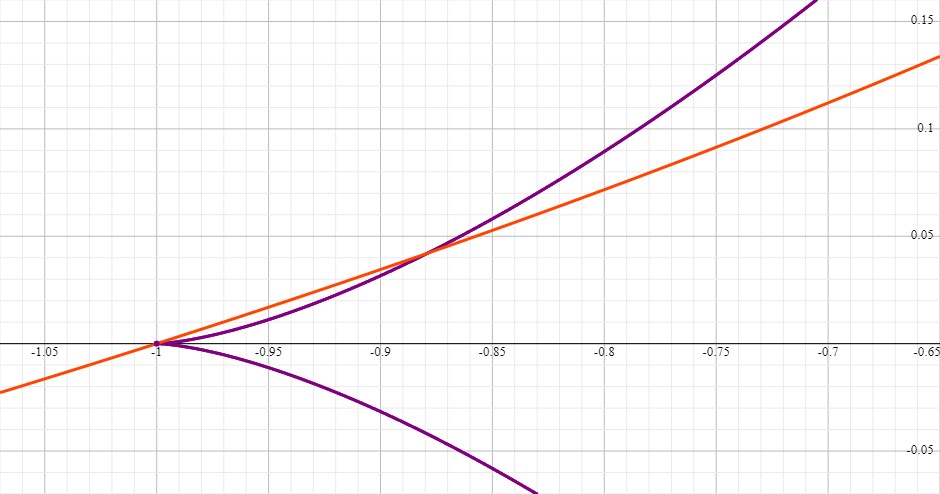
#(0,1)#, #(-1, 0)#, #(0.46622, 1.77541)#, and #(-0.87907, 0.04205)#
The graph below shows these points:
#x=-1# and #x=-0.87907# are very close together and do not show up clearly on the graph. The following is the close up of the area that shows them better: