Question

What is the equation of the tangent line of `f(x)=e^(-x^2)-sin3x` at `x=pi/4`?

Answer 1

`=>y=x(-pi/2e^(-pi^2/16) +3sqrt2/2)+pi^2/8e^(-pi^2/16) -3pisqrt2/8+e^(-pi^2/16)-sqrt2/2`

Explanation:

Your tangent equation at `pi/4` is:
`y-f(pi/4)=f'(pi/4)(x-pi/4)`
so to find the tangent, you need to find `f(pi/4)` and `f'(pi/4)`
Finding `f(pi/4)`:
for `x=pi/4`, `f(pi/4)=e^(-pi^2/16) - sin3pi/4`
`=>f(pi/4)=e^(-pi^2/16) -sqrt2/2`
Finding `f'(pi/4)`:
`f'(x)=-2xe^(-x^2)-3cos3x`
for `x=pi/4`, `f'(pi/4)=-2pi/4e^(-pi^2/16) -3cos3pi/4`
`=> f'(pi/4)=-pi/2e^(-pi^2/16) - 3(-sqrt2/2)`
`=> f'(pi/4)=-pi/2e^(-pi^2/16) + 3sqrt2/2`
After all, you put what you found into your tanget function, so you get:
`y-e^(-pi^2/16) + sqrt2/2 =( -pi/2e^(-pi^2/16) + 3sqrt2/2)(x-pi/4)`
`=>y=x(-pi/2e^(-pi^2/16) +3sqrt2/2)+pi^2/8e^(-pi^2/16) -3pisqrt2/8+e^(-pi^2/16)-sqrt2/2`

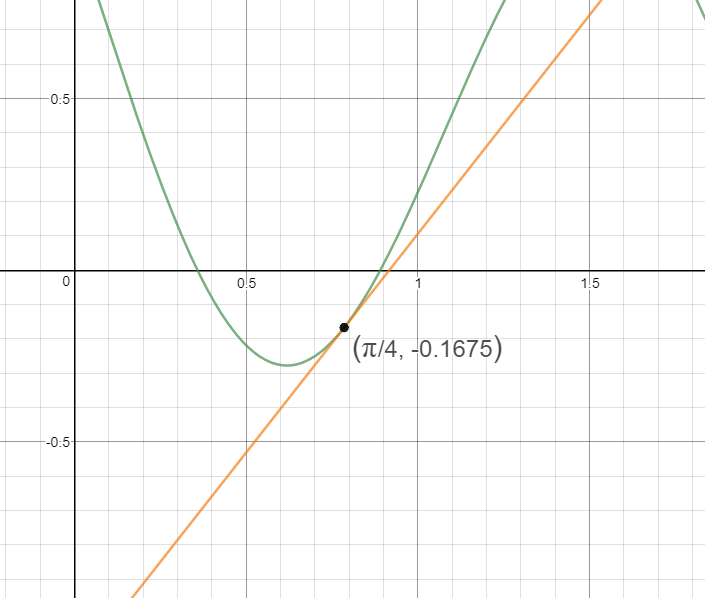
Where the green line is your f(x), yellow line is the tangent of `pi/4`