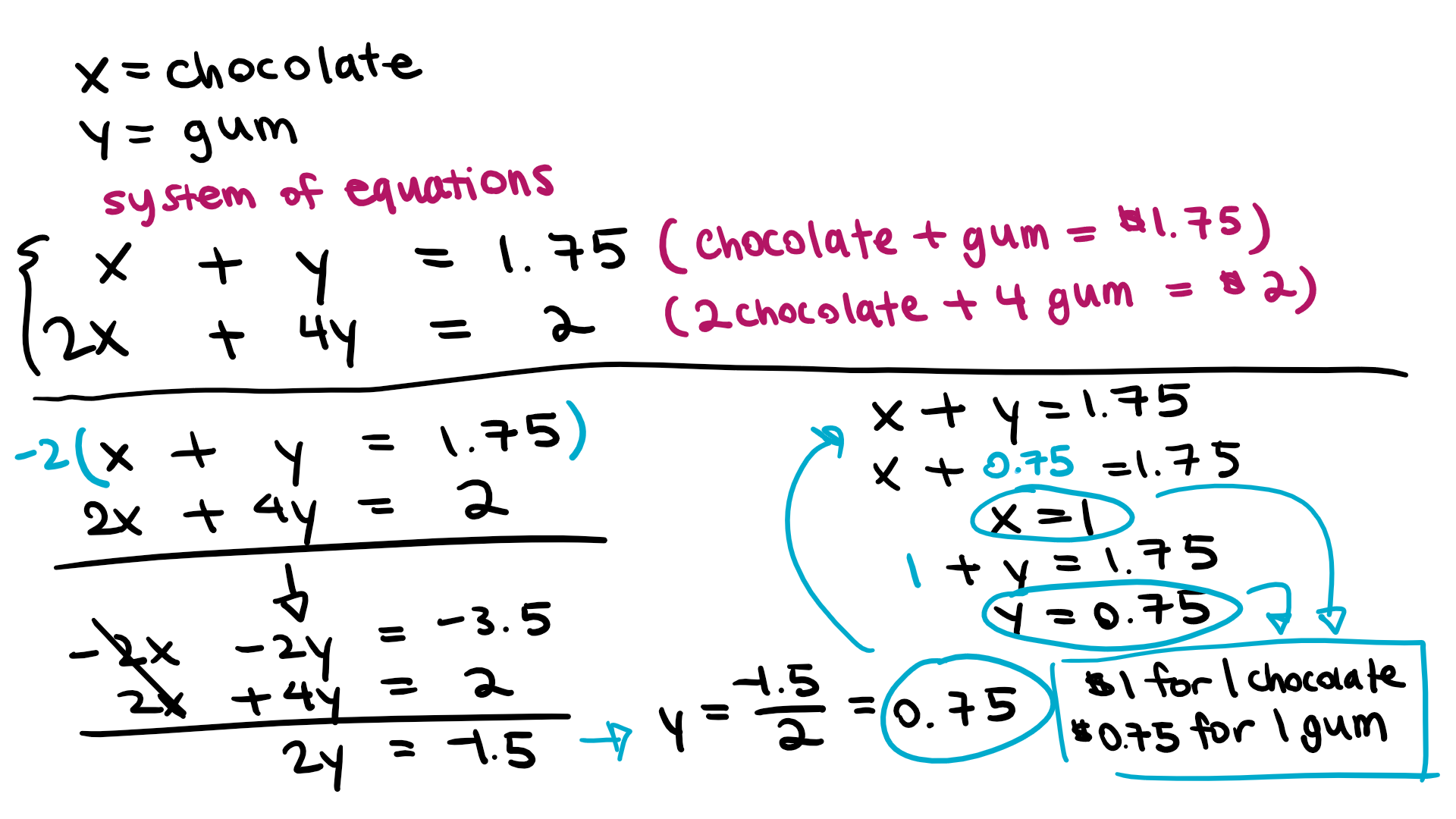Question

Sally bought three chocolate bars and a pack of gum and paid $1.75. Jake bought two chocolate bars and four packs of gum and paid $2.00. Write a system of equations. Solve the system to find the cost of a chocolate bar and the cost of a pack of gum?

Answer 1

Cost of a chocolate bar: $0.50
Cost of a pack of gum: $0.25

Explanation:

Write 2 systems of equations. use `x` for the price of chocolate bars bought and `y` for the price of a pack of gum.
3 chocolate bars and a pack of gum cost $1.75.
`3x + y = 1.75`
Two chocolate bars and four packs of gum cost $2.00
`2x + 4y = 2.00`

Using one of the equations, solve for y in terms of x.
`3x + y = 1.75` (1st equation)
`y = -3x + 1.75` (subtract 3x from both sides)
Now we know the value of y, plug it into the other equation.
`2x + 4(-3x + 1.75) = 2.00`
Distribute and combine like terms.
`2x + (-12x) + 7 = 2.00`
`-10x + 7 = 2`
Subtract 7 from both sides
`-10x = -5`
Divide both sides by -10.
`x = 0.5`
The cost of a chocolate bar is `$0.50`.

Now we know the price of a chocolate bar, plug it back into the first equation.
`3(0.5) + y = 1.75`
`1.5 + y = 1.75` Distribute and combine like terms
`y = 0.25` Subtract 1.5 from both sides.
The cost of a pack of gum is `$0.25`

Answer 2

$1 for 1 chocolate
$0.75 for 1 gum

Explanation:

The set up for the system equations is this:
`x + y = 1.75`
`2x + 4y = 2`
where `x` is chocolate and `y` is gum

To solve the system of equations, we need to solve for the system of equations for the value of one of the variables. To do that, we must manipulate both equations so that one of the variables can be eliminated (in the image below, I chose to eliminate `x`).

After we have one variable (in the image we found the `y` value), we can plug it into ANY of the equations to find the other variable.