Question

What is the horizontal asymptote of `(2x-1)/(x^2-7x+3`?

Answer 1

Please see below.

Explanation:

.

`y=(2x-1)/(x^2-7x+3`

The rule is:

If the degree of the numerator is smaller than the degree of the denominator then the horizontal asymptote is the `x`-axis.

If the degree of the numerator is the same as the degree of the denominator then the horizontal asymptote is `y= ("Coefficient of the highest power term in the numerator")/("Coefficient of the highest power term in the denominator")`

If the degree of the numerator is larger than the degree of the denominator by `1` then there is no horizontal asymptote. Instead the function has a slant asymptote.

In this problem, we have the first case and the horizontal asymptote is the `x`-axis.

If you have learned how to calculate the limits of functions you can calculate the limit of your function as `x->+-oo`. You will see that regardless of which of the three cases your function has, the above rules are correct.

You can see this in the graph of the function below:

Answer 2

`y=0`

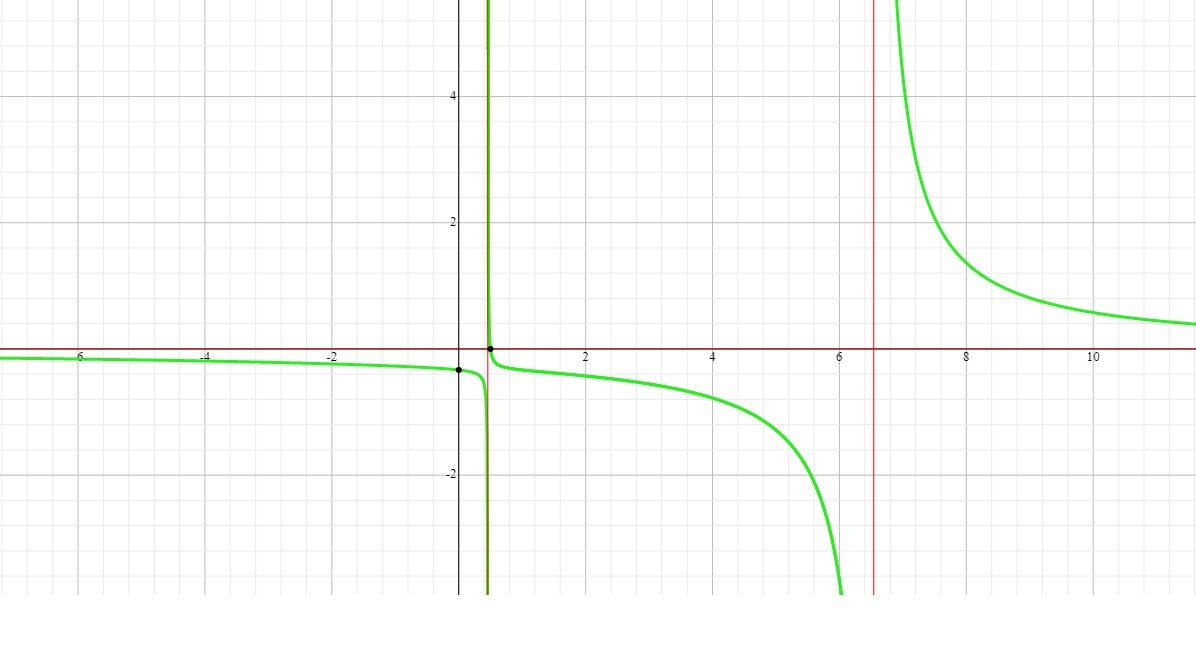
Explanation:

There are 2 ways of doing this.

(1) There is a rule that states that if the polynomial in the numerator has a lower degree than the polynomial in the denominator, then the horizontal asymptote will be `y=0`.

Why?
Well, you can sub in numbers to see that the polynomial with the lesser degree will always have a number less than the polynomial with a greater degree. Since your number in the numerator is smaller than the number in your denominator, when you divide, you will notice that the number approaches 0.

(2) To find the horizontal asymptote, you need to let your equation approach `y ->0`

When you are finding the horizontal asymptote, you divide both the numerator and denominator by the term with the largest degree. ie in this question, you would divide every term by `x^2`

`lim_(y->0) (2x-1)/(x^2-7x+3)`

`lim_(y->0) (2/x-1/x^2)/(1-7/x+3/x^2)`

`lim_(y->0) (0-0)/(1-0+0)`

`lim_(y->0) 0`

Therefore, your horizontal asymptote is `y=0`