Determine the equation of the tangent to f(x) at x=5 ?
Grade 12 calculus
Grade 12 calculus
1 Answer
Please see below.
Explanation:
.
If
Slope of the tangent line to the curve can be calculated by taking the derivative of the function and evaluating it at the point of tangency:
Because the function can only be positive,
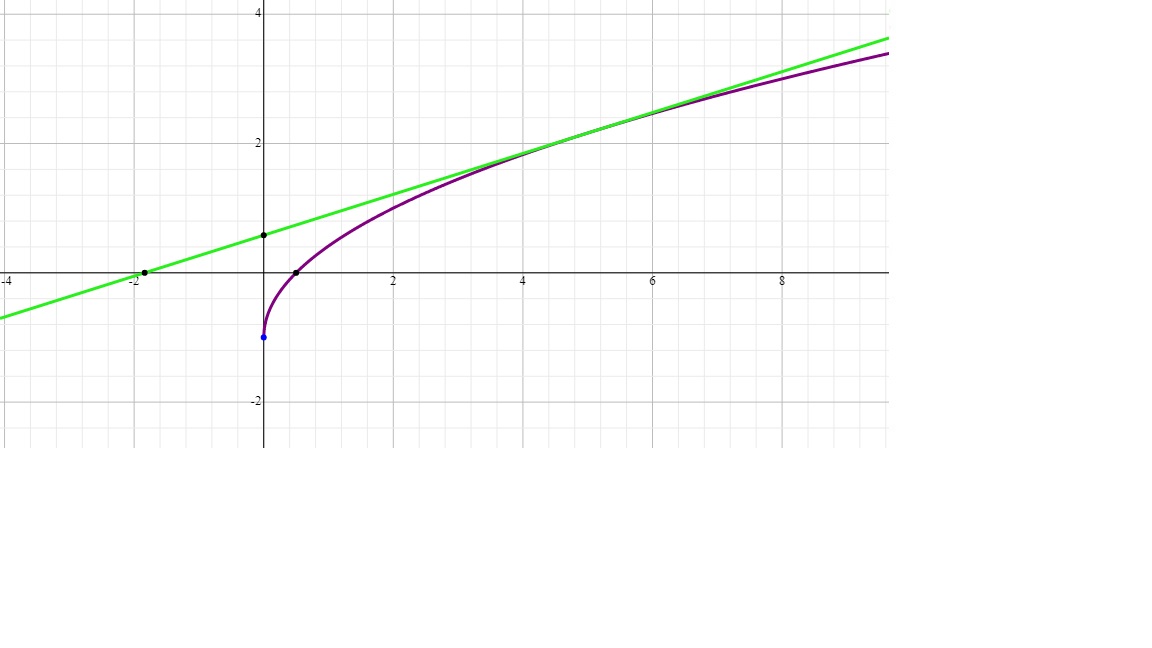
The equation of the tangent line is:
We use the coordinates of the point of tangency to solve for
The equation of the tangent line is:
Here is the graph of the function and its tangent:
If
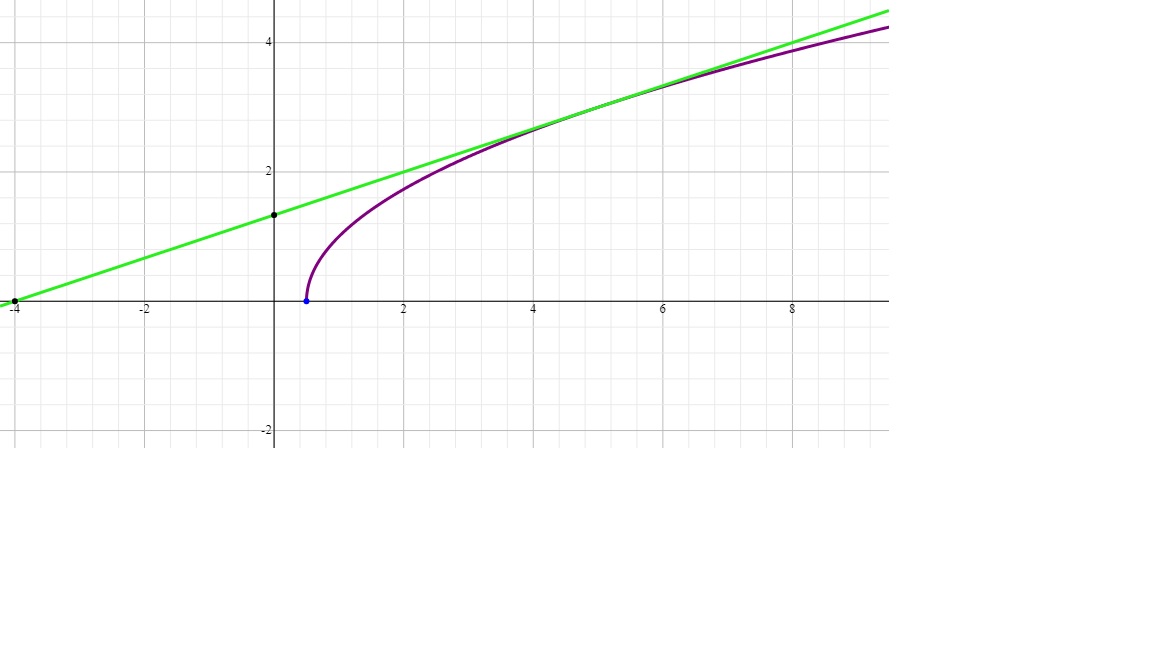
Equation of the tangent line is:
Here is the graph of the function and its tangent: