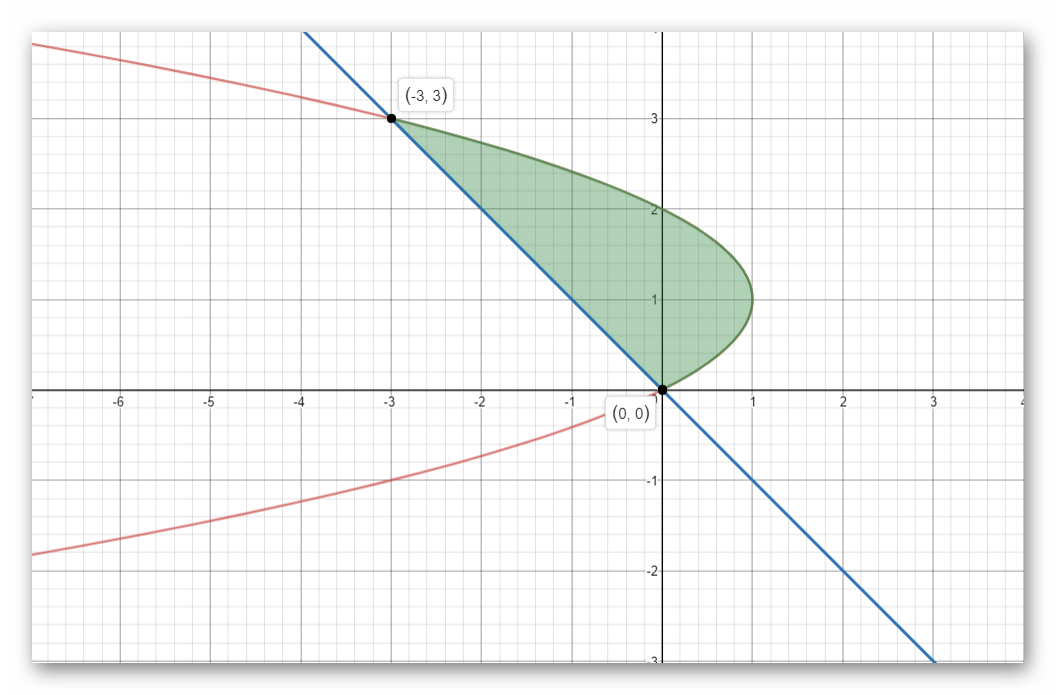
How do you find the area between f(y)=y(2−y),g(y)=−y?
2 Answers
Jun 3, 2018
Explanation:
Claculating the intersection Points:
so we get
So we have
or
and we have
Jun 3, 2018
Explanation:
The area between two curves due to y-axis is given by :
lets find the cross between the curves:
show the wanted area below (shaded):