.
#x^2/9+y^2/4=1#
#x/3+y/2=1#
Let's find the intersection points between the ellipse and the line;
From the equation of the line:
#y/2=1-x/3#
#y^2/4=(1-x/3)^2=1-(2x)/3+x^2/9#
Let's substitute this into the equation of the ellipse:
#x^2/9+x^2/9-(2x)/3+1=1#
#(2x^2)/9-(2x)/3=0#
#x^2/9-x/3=0#
#x^2-3x=0#
#x(x-3)=0#
#x=0,3#
Let's graph the functions:
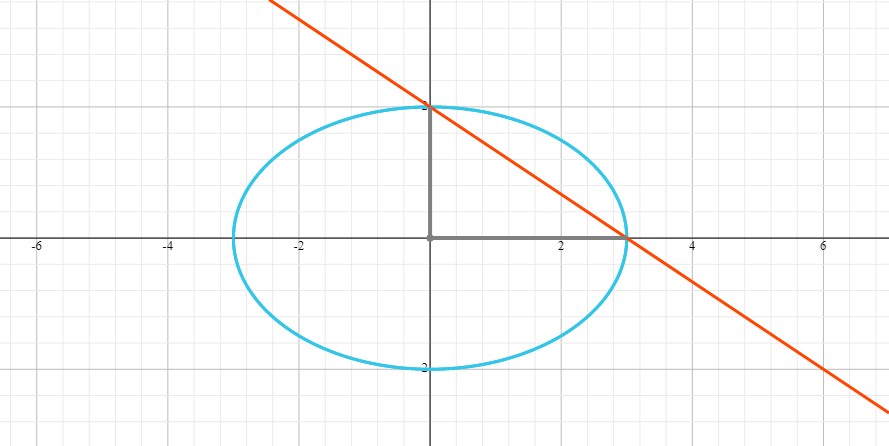
The area of the smaller region bounded by the ellipse and the line can be calculated by taking the integral of a function that is the result of subtracting the lower curve (straight line) from the upper curve (ellipse); and evaluating it between the two values of #x# we just found to be the the intersection points between the curves.
Because we want to integrate along the #x#-axis, we need to find the equations of both curves in the form of #y# as a function of #x#.
#x^2/9+y^2/4=1#
#4x^2+9y^2=36#
#9y^2=36-4x^2#
#y^2=(36-4x^2)/9=(4(9-x^2))/9#
#y=2/3sqrt(9-x^2#
#x/3+y/2=1#
#2x+3y=6#
#3y=6-2x#
#y=(6-2x)/3=(2(3-x))/3#
#A=int_0^3(2/3sqrt(9-x^2)-(2(3-x))/3)dx#
#A=2/3int_0^3(sqrt(9-x^2)-3+x)dx=2/3int_0^3sqrt(9-x^2)dx-2int_0^3dx+2/3int_0^3xdx=2/3I-2x+2/3*1/2x^2)_0^3#
#A=2/3I-2x+1/3x^2)_0^3#
#I=int_0^3sqrt(9-x^2)dx#
Let's figure out #I#. We need to use trigonometric substitution to solve this integral. Let's first construct our right triangle and determine the measures of its sides such that one of them will be #sqrt(9-x^2)#:
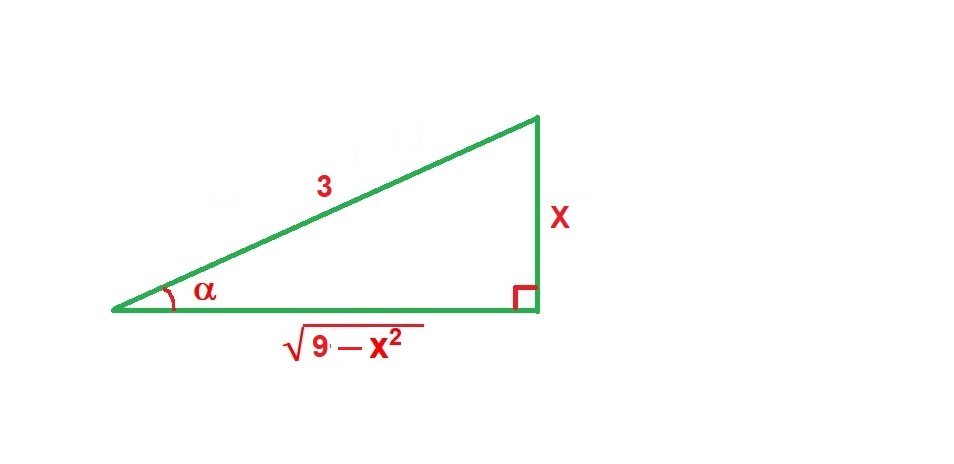
We pick our angle #alpha# and assign #x# as the measure of the opposite side and #3# as the measure of the hypotenuse. Using Pythagoras' formula, we can calculate the measure of the adjacent side:
#3^2=x^2+("Adjacent")^2#
#("Adjacent")^2=9-x^2#
#"Adjacent"=sqrt(9-x^2)#
Now, let's write the three basic trigonometric functions for the angle #alpha#:
#sinalpha=x/3, :. alpha=arcsin(x/3)#
#cosalpha=sqrt(9-x^2)/3#
#tanalpha=x/sqrt(9-x^2)#
From the cosine function, we have:
#sqrt(9-x^2)=3cosalpha#
Taking the derivative of the sine functions, we get:
#cosalphadalpha=dx/3#
#dx=3cosalphadalpha#
Let's substitute these two pieces into our integral argument to turn it into a trigonometric integral:
#I=int(3cosalpha)(3cosalphadalpha)=int9cos^2alphadalpha=9intcos^2alphadalpha#
Let's use a Half-Angle formula to reduce the power of the function:
#I=9int1/2(1+cos2alpha)dalpha=9/2int(1+cos2alpha)dalpha=9/2intdalpha+9/2intcos2alphadalpha=9/2alpha+9/2intcos2alphadalpha=9/2alpha+9/2II#
We will use #u#-substitution to solve the remaining integral:
#u=2alpha#
#du=2dalpha#
#dalpha=(du)/2#
#II=intcosu(du)/2=1/2intcosudu=1/2sinu=1/2sin2alpha#
#I=9/2alpha+9/4sin2alpha#
Now, we can substitute back to turn our function back into algebraic form:
#sin2alpha=2sinalphacosalpha=2(x/3)(sqrt(9-x^2)/3)=2/9xsqrt(9-x^2)#
#I=9/2arcsin(x/3)+1/2xsqrt(9-x^2)#
#A=2/3I-2x+1/3x^2)_0^3#
#A=3arcsin(x/3)+1/3xsqrt(9-x^2)-2x+1/3x^2)_0^3#
#A=3arcsin(1)+0-6+3-(3arcsin(0)+0-0+0)#
#A=3(pi/2)-3-(0)=(3pi)/2-3~~1.7124#



