Could anyone please help me with this question?
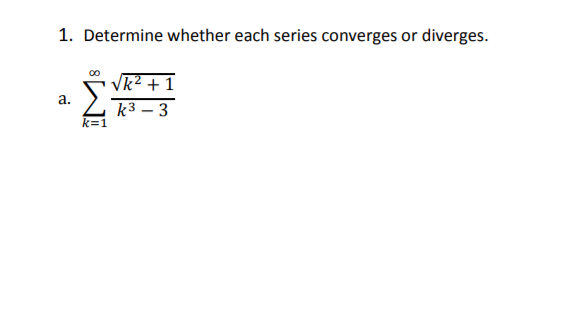
1 Answer
It is converging.
Explanation:
To figure out if a sum of a series of terms converges or diverges we need to decide if the terms are getting bigger or smaller.
In this case the terms are summed for values of
We need to work out if the value of the expression increases as
Looking at the expression we can see that the numerator
It is then going to get closer and closer to k as k increases, so it is increasing at very nearly the same rate as k once k is large.
e.g. at
which is already pretty close to
The denominator is 3 less than
e.g. at
So we see that as
So it is clearly converging.

