Question #f4fb1
1 Answer
Feb 9, 2017
 mathworld.wolfram.com
mathworld.wolfram.com
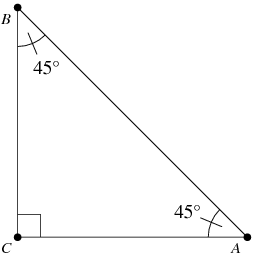
Let us consider a right angled isosceles triangle ABC inwhich
So by Pythgorean theorem
Again
So
 mathworld.wolfram.com
mathworld.wolfram.com
Let us consider a right angled isosceles triangle ABC inwhich
So by Pythgorean theorem
Again
So

