Question

A circle cuts the parabola `y^2=4ax` in the points `(at_i^2, 2at_i)` for `i=1, 2, 3, 4`. Prove that `t_1+t_2+t_3+t_4=0`?

Answer 1

Please see below.

Explanation:

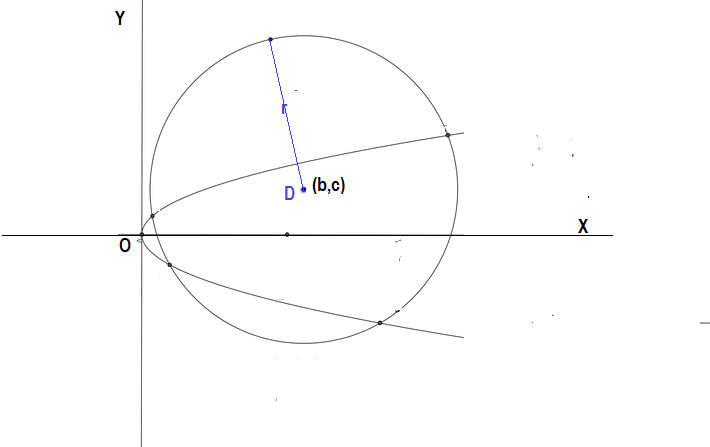
As the parametric form of equation of parabola `y^2=4ax` is `(at^2,2at)` all the points `(at_i^2,2at_i)` for `i=1,2,3,4.` too lie on the parabola.

As they are points of intersection of parabola and circle, they also fall on the circle i.e. `(at_i^2,2at_i)` for `i=1,2,3,4.` lie on circle. Let these points be `T_1,T_2,T_3,T_4` respectively in cyclical order, as shown in the figure below.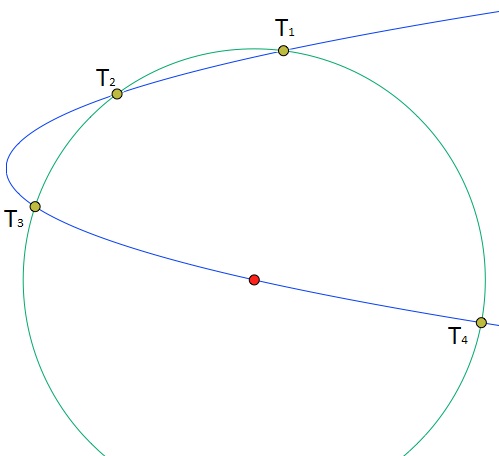

Now `T_1T_2T_3T_4` is a cyclical quadrilateral and hence

`m/_T_2T_1T_4+m/_T_2T_3T_4=180^@` and `tanT_1=-tanT_3`(A)

To find `m/_T_2T_1T_4`, let us find the slopes of `T_2T_1` and `T_2T_4`.

Slope of `T_2T_1`, joining `(at_2^2,2at_2)` and `(at_1^2,2at_1)` is

`(2a(t_2-t_1))/(a(t_2^2-t_1^2))=2/(t_2+t_1)`

and similarly slope of `T_1T_4`, joining `(at_1^2,2at_1)` and `(at_4^2,2at_4)` is

`(2a(t_1-t_4))/(a(t_1^2-t_4^2))=2/(t_1+t_4)`

Now as measure of angle between two lines with slope `m_1` and `m_2` is `tan^(-1)((m_1-m_2)/(1+m_1m_2))`, we have

`tanT_1=(2/(t_2+t_1)-2/(t_1+t_4))/((1+(2/(t_2+t_1))xx(2/(t_1+t_4)))`

= `(2(t_1+t_4-t_2-t_1))/((t_1+t_4)(t_2+t_1)+4)=(2(t_4-t_2))/((t_1+t_4)(t_2+t_1)+4)`

Similarly `tanT_3=(2/(t_4+t_3)-2/(t_3+t_2))/((1+(2/(t_4+t_3))xx(2/(t_3+t_2)))`

= `(2(t_3+t_2-t_4-t_3))/((t_4+t_3)(t_3+t_2)+4)=(2(t_2-t_4))/((t_4+t_3)(t_3+t_2)+4)`

and using (A) we get

`(2(t_4-t_2))/((t_1+t_4)(t_2+t_1)+4)=-(2(t_2-t_4))/((t_4+t_3)(t_3+t_2)+4)`

or `(t_1+t_4)(t_2+t_1)+color(red)4=(t_4+t_3)(t_3+t_2)+color(red)4`

or `t_1t_2+t_1^2+color(red)(t_4t_2)+t_4t_1=t_4t_3+color(red)(t_4t_2)+t_3^2+t_3t_2`

or `t_1t_2+t_1^2+t_4t_1-t_4t_3-t_3^2-t_3t_2=0`

or `t_2(t_1-t_3)+t_4(t_1-t_3)+(t_1+t_3)(t_1-t_3)=0`

and dividing by `(t_1-t_3)`, we get

`t_1+t_2+t_3+t_4=0`

Answer 2

Let the equation of the circle which cuts the given parabola `y^2=4ax` be `(x-b)^2+(y-c)^2=r^2.`,where `(b,c)` is the coordinates of the center(D) of the circle and `r` is its radius.

Replacing `x=y^2/(4a)` in the equation of the circle we get the following equation of y of degree 4

`(x-b)^2+(y-c)^2=r^2.`

`=>x^2-2bx+b^2+y^2-2cy+c^2-r^2=0`

`=>(y^2/(4a))^2-2b(y^2/(4a))+b^2+y^2-2cy+c^2-r^2=0`

`=>y^4/(16a^2)+(1-(2b)/(4a))y^2-2cy+b^2+c^2-r^2=0color(red)(.....[1])`

Here `f(y)=0` is an equation of y of degree 4. So it will have 4 roots of y and those roots will be given by `2at_i" for " i=1,2,3,4`

Since the coefficient of `y^3` of equation [1] is zero , the sum of all 4 roots of y must be zero.

Hence `sum_(i=1)^(i=4)(2at_1)=0`

`=>sum_(i=1)^(i=4)t_1=0`

`=>t_1+t_2+t_3+t_4=0`