A triangle has sides A, B, and C. Sides A and B are of lengths #2# and #8#, respectively, and the angle between A and B is #pi/3#. What is the length of side C?

Question

1257 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-01-10T10:44:25

#C=2sqrt(13)#

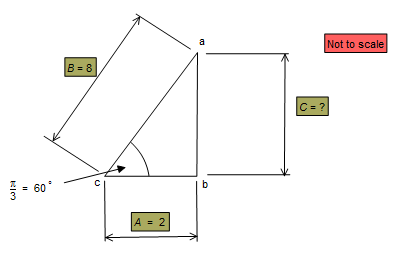
enter image source here

#color(blue)("Test if "/_b = pi/2 -> 90^0#

Known that #cos(pi/3)=1/2#

If and only if ( iff ) #/_b = pi/2# then #A/B-> 1/2#
But #A/B = 2/8 = 1/4 => /_b != pi/2#

#color(blue)("Shown that "/_b !=pi/2#
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~

#color(blue)("Solve using the Cosine rule")#

#C^2 = A^2+B^2 -2ABcos(c)#

#=> C^2=2^2+8^2 -2(2)(8)cos(pi/3)#

#C^2=4+64-32(1/2)#

#C^2= 52#

#C=2sqrt(13)#