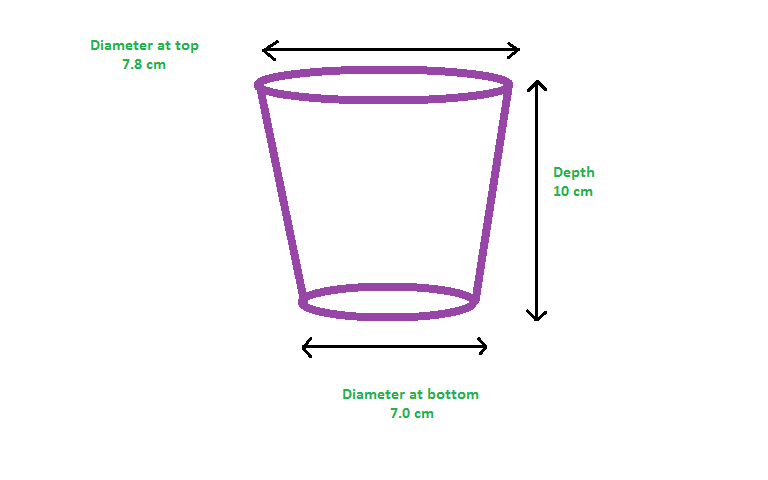
At which depth will the glass, which is it not a right cylinder, be volumetrically half-filled? Assume that the thickness of the base is negligible and the thickness of the walls is constant.

I think I would first have to find the derivative of perhaps the circular area or something like that... Out of the box answers are welcome:))
The glass.

1 Answer
Steve M
Jan 28, 2018

The split should occur at a height of approx #5.27 \ cm#

Explanation:

No Calculus required:

The mathematical name for the given "bucket" , or "glass" shape is a frustum, we can readily calculate the volume using the formula:

# V = pi/3(R^2 + rR + r^2)h \ \ \ # (See Notes)

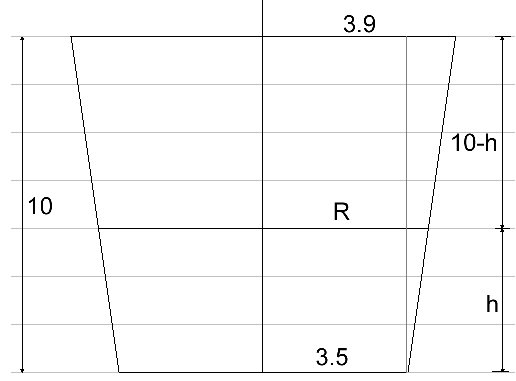
We are required to split the volume of the given frustum of lower radius #3.5 \ cm#, upper radius #3.9 \ cm# and height #10 \ cm# into two further frustums, each having equal volume.

Steve M

Suppose the volumeatic split occurs at a height #h \ cm# so that the lower frustum is of height #h#, with lower radius #3.5 \ cm# upper radius #R#. Then the upper frustum will be of height #10-h# with lower radius #r# and upper radius of #3.9 \ cm#.

Using this information, and utilising the frustum formula, we can write the identical volume of each frustum as:

# pi/3(3.5^2 + 3.5R + R^2)h = pi/3(R^2 + 3.9R + 3.9^2)(10-h) #

And by similar #triangle#'s:

# h : (R-3.5) = 10: (3.9-3.5) #

# :. h/(R-3.5) = 10/(0.4) #
# :. 0.4h = 10(R-3.5) #
# :. 0.4h = 10R-35 #
# :. 10R = 0.4h +35 #

Then we solve the two equations simultaneously, which results in the solution:

# h ~~ 5.26948# #R~~3.71078# (5dp)

|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||

Notes:
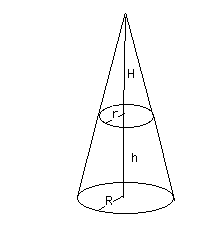
We can readily derive the frustum volume by considering the difference in the volumes of standard cones#

http://jwilson.coe.uga.edu/

Using the standard volume of a cone, V=1/3pir^2h; we have:

Volume of Smaller cone:

# V_1 = 1/3pir^2H #

Volume of Larger cone:

# V_2 = 1/3piR^2(H+h) #

So the volume of the frustum is:

# V = V_2-V_1 #
# \ \ \ = 1/3piR^2(H+h) - 1/3pir^2H #
# \ \ \ = 1/3pi(R^2H+R^2h-r^2H) #
# \ \ \ = 1/3pi(H(R^2-r^2)+R^2h) #
# \ \ \ = 1/3pi(H(R+r)(R-r)+R^2h) #

And by similar #triangle#'s:

# R : H+h = r : H #

# :. R/(H+h) =r/H #
# :. RH = r(H+h) #
# :. RH = rH+rh #
# :. H(R-r) = rh #
# :. H = (rh)/(R-r) #

Substituting into out volume formula we get:

# V = 1/3pi((rh)/(R-r)(R+r)(R-r)+R^2h) #
# \ \ \ = 1/3pih((r)(R+r)+R^2) #
# \ \ \ = 1/3pi(rR+r^2+R^2) # QED

Related questions
Impact of this question
2202 views around the world
You can reuse this answer
Creative Commons License