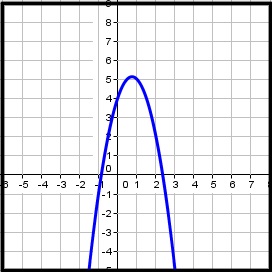
Between what two points are the solutions to the quadratic equation whose related quadratic function is graphed below?

enter image source here

2 Answers
Jumbotron
Jan 18, 2018

Please see the process steps below;

Explanation:

From the Image in the link above the question, it is noted that the roots are;#-1 and 2.2# respectively..

We know that a quadratic equation will be in the form;

#y = ax^2 + bx + c#

We can use the given roots above to denote the quadratic equation using the steps below;

#x = - 1 or x = 2.2 = 11/5#

#x + 1= 0 or x - 11/5 = 0#

#(x + 1) (x - 11/5)#

Expanding the bracket..

#x (x - 11/5) + 1 (x - 11/5)#

#x^2 - (11x)/5 + x - 11/5#

Multiply through by the LCM, in this case the LCM is #5#

#5 (x^2) - 5 (11x)/5 + 5 (x) - 5 (11/5)#

#5x^2 - cancel5 (11x)/cancel5 + 5x - cancel5 (11/cancel5)#

#5x^2 - 11x + 5x - 11#

Simplifying..

#5x^2 - 6x - 11 -> "Quadratic Equation"#

#y = 5x^2 - 6x - 11 -> "Quadratic Graph"#

Jim G.
Jan 19, 2018

#"see explanation"#

Explanation:

#"the solution to any quadratic equation are the values of x"#
#"that make the equation equal zero"#

#"graphically these are the values of x where the graph"#
#"crosses the x-axis"#

#"from the given graph these are"#

#"x between - 1 and zero and x between 2 and 3"#

#"this can be expressed in the following way"#

#-1< x < 0" or "2 < x <3#

Related questions
Impact of this question
2819 views around the world
You can reuse this answer
Creative Commons License