Given a conicoid
#C->f(p) = 0# with #p = (x,y,z)#
and a line
#L->p=p_0 + lambda vec v#
the plane #Pi# tangent to #C# at #p = p_t# has the equation
#Pi-> < vec n_t, p_t - p > = 0#
where #< cdot, cdot ># represents the scalar product of two vectors
and #vec n_t# is the normal vector to #C# at point #p_t#
Now, if #Pi# contains the line #L# then
#Pi @ L = 0# or
#< vec n_t, p_t - p_0-lambda vec v > = 0# or
#< vec n_t, p_t-p_0> - lambda < vec n_t, vec v > = 0#
this must be observed for all #lambda in RR# so we need
#< vec n_t, vec v > = 0#
Resuming, the tangency with the condition #Pi @ L = 0# can be reduced to
#{(f(p_t)=0),(<< vec n_t,p_t-p_0 >> = 0),(<< vec n_t, vec v >> = 0):}#
Three equations with three unknowns: #p_t=(x_t,y_t,z_t)#
In our case we have
#vec n_t = (14 x_t, -6 y_t, -2 z_t)#
#p_0 = (0,3/2,3)# and
#vec v =(6,7,0) #
So solving
#{(21 + 7 x_t^2 - 3 y_t^2 - z_t^2 = 0),
(14 x_t^2 - 6 (y_t-3/2) y_t - 2 (z_t-3) z_t = 0), (84 x_t - 42 y_t = 0):}#
and the solutions
#p_(t_1)= (1,2,4)# and
#p_(t_2)=(2,4,1)#
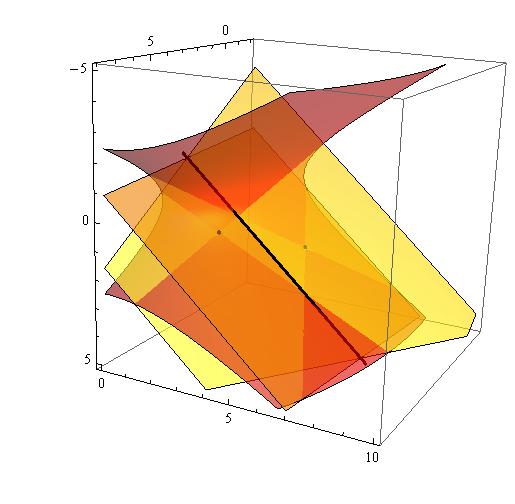
Attached a plot showing the arrangement.