Find the equation of the tangent to the curve #x + xy + y = 5# at #x = 5# help?

2 Answers
Noah G
Jan 10, 2018

#y = 5/6 - 1/6x#

Explanation:

Start by finding the y-value:

#5 + 5y + y = 5#

#6y = 0#

#y = 0#

Now we find the derivative using implicit differentiation.

#1 + y + x(dy/dx) + dy/dx = 0#

#y + x(dy/dx) + dy/dx = -1#

#x(dy/dx) + dy/dx = -1 - y#

#dy/dx(x + 1) = -1 - y#

#dy/dx = (-1 - y)/(x + 1)#

#dy/dx= -(y + 1)/(x + 1)#

At #(5, 0)#, the derivative will have value

#dy/dx= -1/6#

Now use point-slope form to find the equation:

#y - y_1 = m(x - x_1)#

#y - 0 = -1/6(x - 5)#

#y = -1/6x +5/6#

Hopefully this helps!

Douglas K.
Jan 10, 2018

#y = -1/6x+5/6#

Explanation:

Begin by finding the y coordinate at #x = 5#:

#5+5y+y=5#

#5y+y = 0#

#6y = 0#

#y = 0#

The point of tangency is #(5,0)#

Compute the first derivative of the curve:

#1+y+xdy/dx+dy/dx =0#

#(x+1)dy/dx = -(y+1)#

#dy/dx = -(y+1)/(x+1)#

The slope, m, of the tangent line is the first derivative evaluated at the point #(5,0)#:

#m = -(0+1)/(5+1)#

#m = -1/6#

Use the point-slope form for the equation of a line:

#y = m(x-x_0)+y_0#

#y = -1/6(x-5)+0#

#y = -1/6x+5/6#

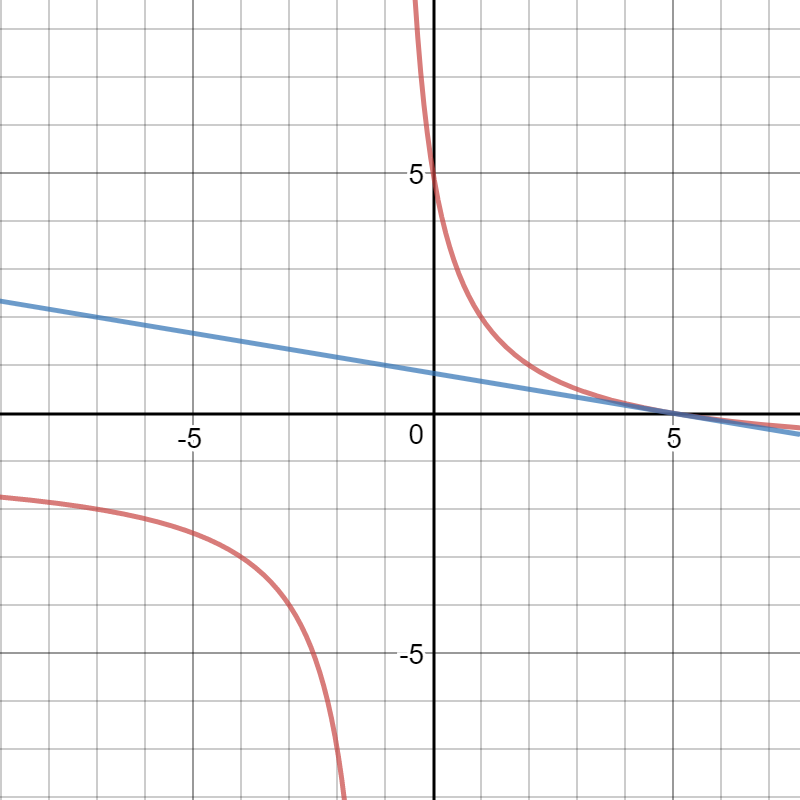
Here is the a graph of the curve and the tangent line:

www.desmos.com/calculator

Related questions
Impact of this question
1330 views around the world
You can reuse this answer
Creative Commons License