Finding #DeltaH# given heat released and mass in bomb calorimetry?
When 8.23 g of the organic compound cinnamaldehyde, C9H8O, was burned in a bomb calorimeter at 298K, the heat released was 302.9 kJ. Calculate the enthalpy of combustion of cinnamaldehyde at this temperature.
When 8.23 g of the organic compound cinnamaldehyde, C9H8O, was burned in a bomb calorimeter at 298K, the heat released was 302.9 kJ. Calculate the enthalpy of combustion of cinnamaldehyde at this temperature.
1 Answer
#DeltaH_C = -"4870 kJ/mol"# to 3 sig figs.
Note that you are using a bomb calorimeter. That is a constant-volume system, not a constant-pressure system.
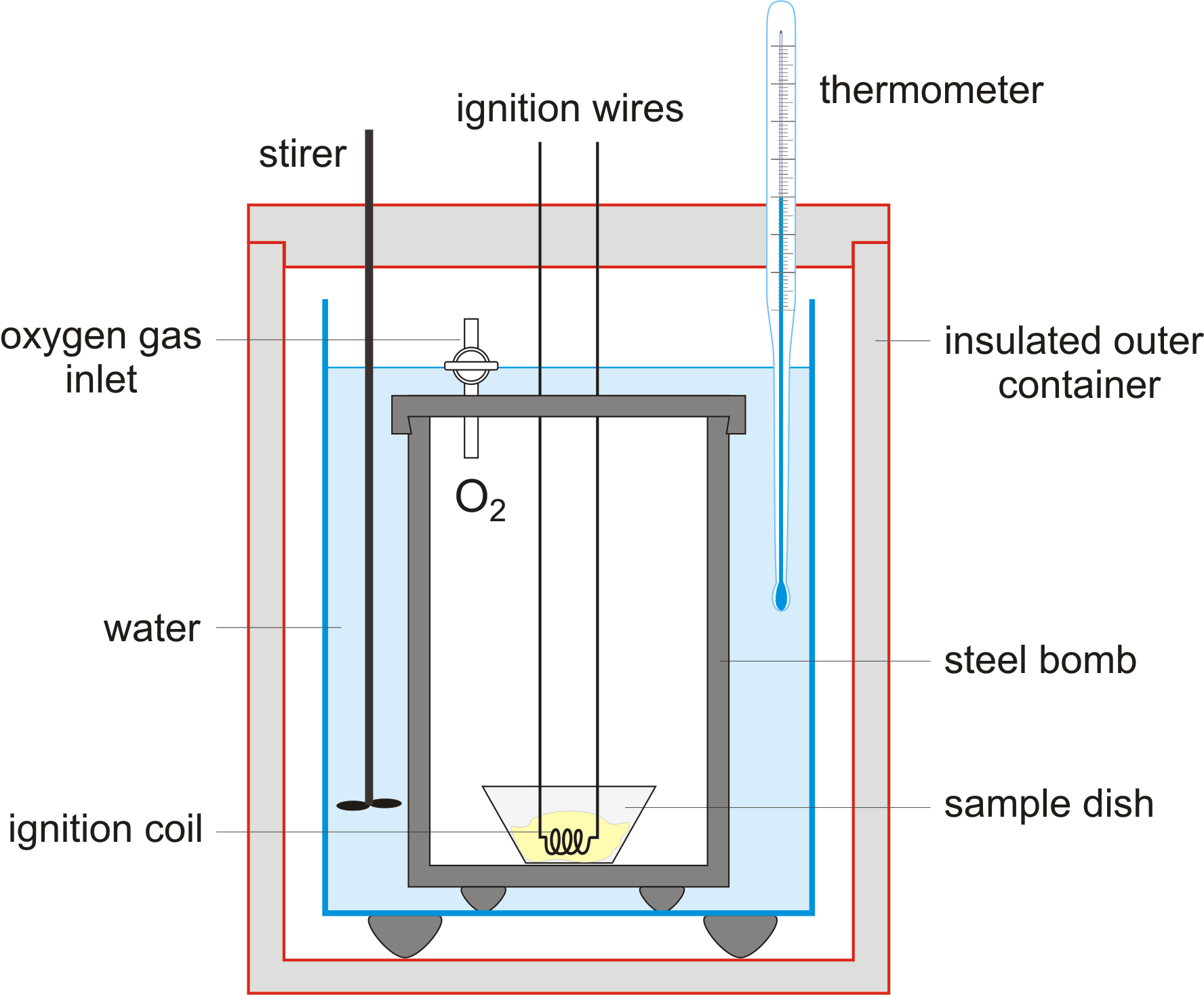
That adds a complication, in that for a thermodynamically-closed system,
#color(green)(DeltaH_C = DeltaE_C + Delta(PV)_C)# #" "bb((1))# where
#DeltaE_C# is the change in internal energy of combustion and#Delta(PV)_C# is the change in enthalpy due to pressure-volume work.
At constant volume,
The balanced reaction is:
#"C"_9"H"_8"O"(s) + 21/2"O"_2(g) -> 9"CO"_2(g) + 4"H"_2"O"(l)# where we assume the water to be a liquid in a closed rigid container, having condensed down from initially forming a gas.
For your scale of reaction, you have
#8.23 cancel"g" xx "1 mol"/(132.16 cancel"g cinnamaldehyde") = "0.0623 mols"#
For ideal gases,
#DeltaH_C = DeltaE_C + Delta(nRT)# .
The temperature of the surroundings (water + room) is typically constant in a bomb calorimetry experiment, as it is done in a huge, probably
Thus,
#DeltaH_C ~~ DeltaE_C + Deltan cdot RT_"room"#
For the reaction as-written, the change in mols of gas dominates the pressure-volume work due to the combustion. So we take:
#DeltaH_C ~~ DeltaE_C + Deltan_"gas" cdot RT_"room"# #" "bb((2))#
and get (
#Deltan_"gas" = 9 - 21/2 = -"1.5 mols"#
On the scale of the actual reaction, we have only
#Deltan_"gas" = -"1.5 mols" xx "0.0623 mols cinnamaldehyde used"/"1 mol cinnamaldehyde as-written"#
#= -"0.0935 mols gas"#
We now have enough info. Using
#DeltaH_C = -"302.9 kJ" + (-0.0935 cancel"mols gas" cdot "0.008314472 kJ/"cancel"mol"cdotcancel"K" cdot 298.15 cancel"K")#
#= -"303.13 kJ"#
And in terms of
#color(blue)(DeltaH_C) = -"303.13 kJ"/(8.23 cancel"g") xx (132.16 cancel"g")/"1 mol"#
#= color(blue)(-"4870 kJ/mol")#
to 3 sig figs.

