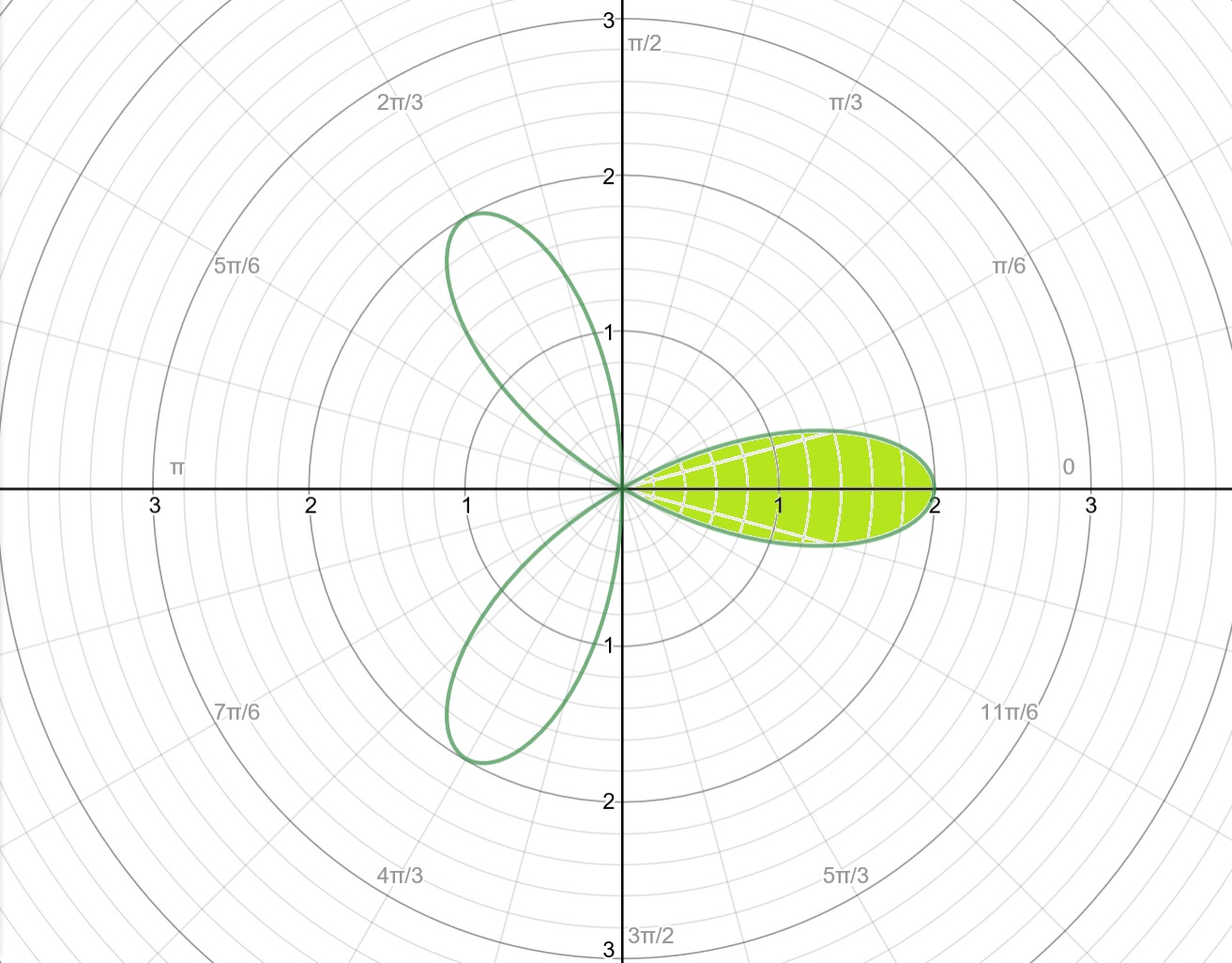
First, graph r=2cos(3theta) to get an idea of what the petals look like. It can be really helpful to draw concentric circles and radial angle lines on graph paper, so that you have a polar graph, like this:
 )
)
Next, using either a graphing utility or this graph paper, plot the graph using convenient points.
{:(theta,r),(--,--),(0,2),(+-pi/12,+-sqrt(2)~~+-1.41),(+-pi/8,+-sqrt(2-sqrt(2))~~+-0.77),(+-pi/6,0):}
 )
)
The area of a petal can be determined by an integral of the form
A=1/2int_alpha^betar(theta)^2 d theta
Notice the petal in Quadrant I and IV does not extend past +-pi/6 and that it is perfectly split between the two quadrants. That implies that if we can find the are of just half a petal, then we can multiply the result by two and get the area of the entire petal.
Letting the interval of integration go from theta=0 to theta=pi/6 and doubling the entire integration gives
A=2xx1/2int_0^(pi/6)4cos^2(3theta)d theta
A=4int_0^(pi/6)cos^2(3theta)d theta
The half-angle identity for cosine says
cos^2(u)=(1+cos(2u))/2, so
cos^2(3theta)=(1+cos(6theta))/2.
This changes our integral to
A=4int_0^(pi/6)(1+cos(6theta))/2d theta
A=2int_0^(pi/6)(1+cos(6theta))d theta
A=2int_0^(pi/6)d theta+2int_0^(pi/6)cos(6theta)d theta
Using u-substitution, you can determine the integral of the cosine function.
A=2[theta]_0^(pi/6)+2[1/6sin(6theta)]_0^(pi/6)
A=pi/3
 )
)  )
) 