Question

How do you find the instantaneous rate of change at a point on a graph?

Answer 1

The instantaneous rate of change at a point is equal to the function's derivative evaluated at that point. In other words, it is equal to the slope of the line tangent to the curve at that point.

For example, let's say we have a function `f(x) = x^2`.

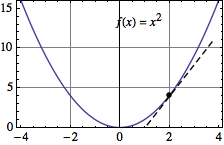
If we want to know the instantaneous rate of change at the point `(2, 4)`, then we first find the derivative:

`f'(x) = 2x`

And then we evaluate it at the point `(2, 4)`:

`f'(2) = 2*2 = 4`

So, the instantaneous rate of change, in this case, would be `4`.