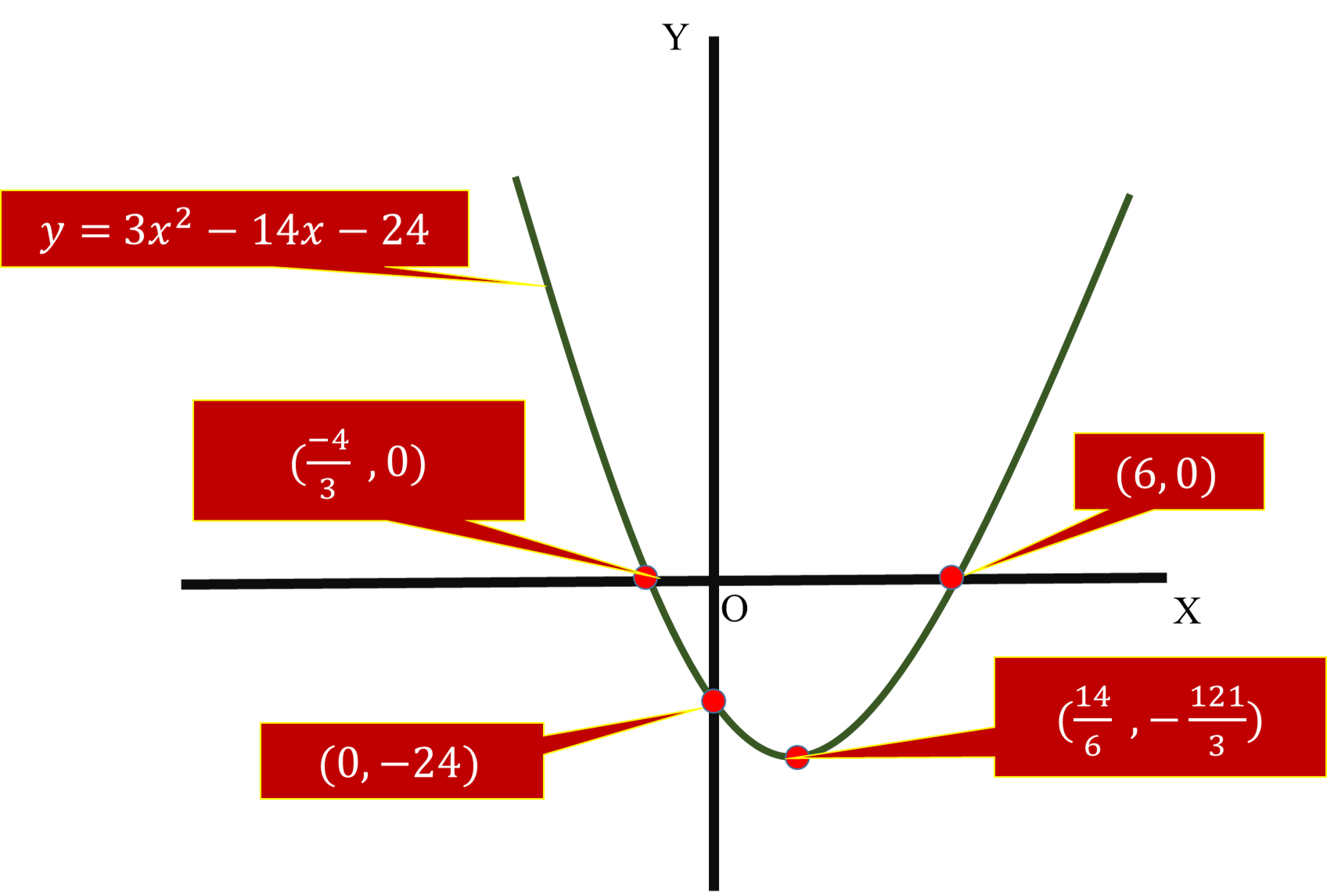
How do you find the vertex and the intercepts for #f(x) = 3 x^2 − 14 x − 24#?
1 Answer
Aug 28, 2016
Vertex
Y - intercept
One of the x-intercept
Another x-intercept
Explanation:
Given -
#y=3x^2-14x-24#
Find the Vertex
x-coordinate of the vertex
#x=(-b)/(2a)=(-(-14))/(2xx3)=14/6#
y-coordinate of the vertex
#y=3(14/6)^2-14(14/6)-24=-121/3#
Vertex
Y - intercept
At
X - intercepts
At
#3x^2-14x-24=0#
#x=((-b)+-sqrt(b^2-(4ac)))/(2a)=(-(-14)+-sqrt((-14)^2-(4xx3xx(-24))))/(2xx3)#
#x=(b+-sqrt(196 -(-288)))/6=(14+-22)/6#
#x=(14+22)/6=6#
One of the x-intercept
#x=(14-22)/6=(-8)/6=(-4)/3#
Another x-intercept