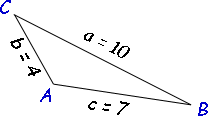First, though, here's a diagram.
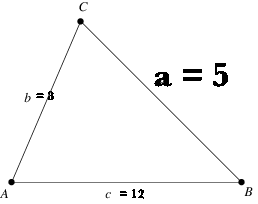
The formula for the Law of Cosine's is the following:
#cosA = (b^2 + c^2 - a^2)/(2bc)#
#cosA = (8^2 + 12^2 - 5^2)/(2 xx 8 xx 12)#
#cosA = 0.953125#
#A = cos^-1(0.953125)#
#A ~~ 17.61˚ or 0.39# radians
Now for angle B:
#cosB = (a^2 + c^2 - b^2)/(2ac)#
#cosB = (5^2 + 12^2 - 8^2)/(2 xx 5 xx 12)#
#cosB = 0.875#
#B = cos^-1(0.875)#
#B ~~28.96˚ or 0.51# radians
Finally for angle C:
#cosC = (a^2 + b^2 - c^2)/(2ab)#
#cosC = (5^2 + 8^2 - 12^2)/(2 xx 5 xx 8)#
#cosC = -0.6875#
#C = cos^-1(-0.6875)#
#C = 133.43˚ or 2.33# radians
Practice exercises:
- Solve the following triangles using the Law of Cosine's.
a)
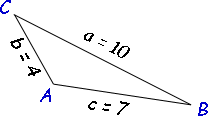
b) 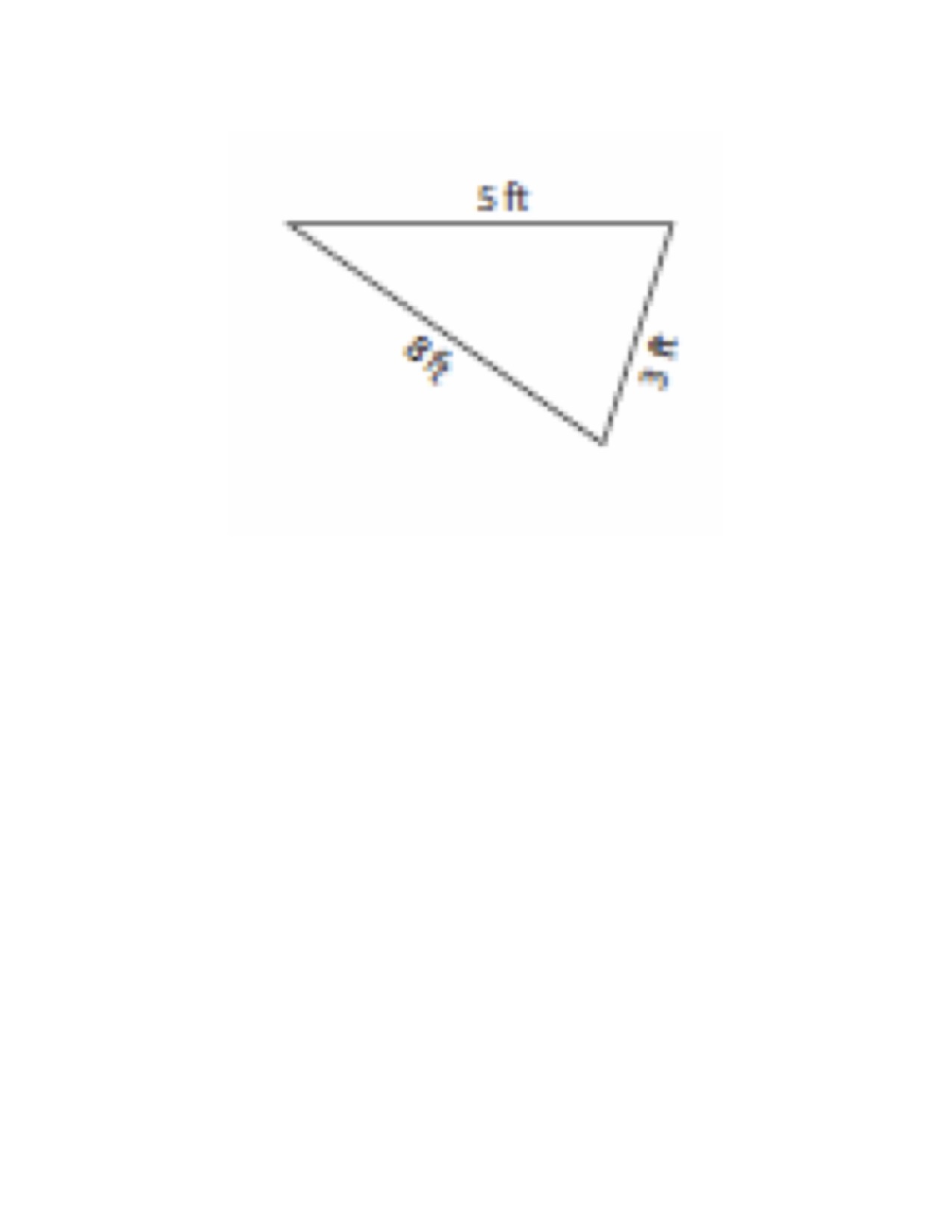
c) #a = 14, b = 15, c = 16#
#2.# We use the formula #a^2 = b^2 + c^2 - (2bc xx cosA)#, a variation on the Law of Cosine's to find a missing side in a triangle. Use this formula to solve the following triangle:
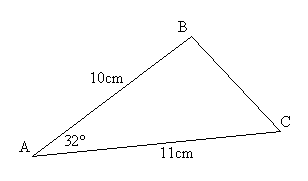
Hopefully this helps, and good luck!