Question

How do you solve the triangle given ∠B = 151°, ∠C = 7°, a = 31?

Answer 1

b= 40.12, c=10.09 and `angle A`= `22^o`

Explanation:

The triangle would appear to like in the figure given below. Angle A would be 180- (151+7) = `22^o`

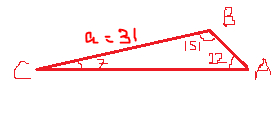
Using sine formula,

`sin22 /31 = sin 151 /b = sin 7 /c`

b= `31* sin 151 /sin22`= 40.12

c= `31* sin7 / sin 22` =10.09

Answer 2

Subtract `angle B and angle C` from `180^@`, to find `angle A`, then use The Law of Sines to find the lengths of sides "b" and "c".

Explanation:

`angle A = 180^@ - angle B - angle C`

`angle A = 180^@ - 151^@ - 7^@`

`angle A = 22^@`

Use The Law of Sines

`a/sin(A) = b/sin(B) = c/sin(C)`

`b = asin(B)/sin(A)`

`b = 31sin(151^@)/sin(22^@)`

`b~~ 40`

`c = asin(C)/sin(A)`

`c = 31sin(7^@)/sin(22^@)`

`c~~ 10`