How do you use the law of sines to solve the triangle given C = 67°, a = 21, c = 20?
1 Answer
May 4, 2015
Given two sides of a triangle and a non-contained angle on the longer side, two different triangles are possible.
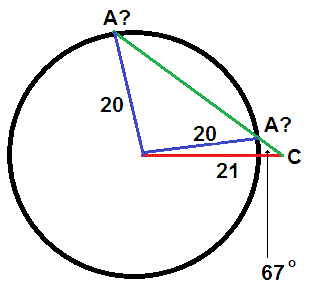
Casual application of the Sine Law will only give you one of these.
unless you are very careful.
By the Sine Law
According to Excel (and I assume any standard calculator)
That is
BUT
So A could be (approx.)
or A could be (approx.)
and you will need to calculate the third angle and side separately for each of these possibilities
