If a baseball player can throw a ball at 45° to a point 100m away horizontally to the initial vertical level, how high could he throw it vertically upward?
1 Answer
Explanation:
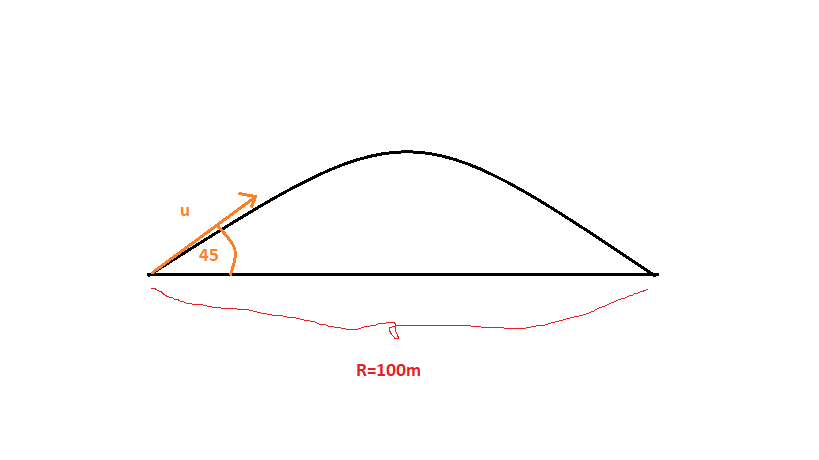
The given information means,he can throw the ball with maximum velocity of
Range of a projectile motion is given as,
Here,
So, we get,
Now,if he would have thrown the ball with this velocity upwards,if it would have reached a maximum distance of
Then using
here,
So,
Derivation of formula of range of projectile motion.
Suppose,the projectile projected with a velocity of
And,from vertical motion we can say,if it reaches the maximum height in time
Now,the projectile will take same time to fall down, So,
So,putting this value of

