If a liquid from a completely filled spherical container of inner radius #r# is poured into a cube shaped container, what would be the dimensions of the cube in terms of the radius of the sphere?

Question

3266 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-09-28T10:09:15

#root(3)(36pi)/3r#

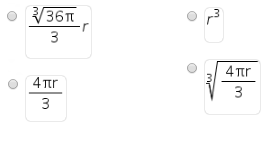
Volume of cube is equal to volume of sphere.

Volume of a sphere: #V = 4/3pir^3#

Let #a# be a side of the cube.

Then:

#a^3# = volume of cube.

So we have:

Volume of cube = volume of sphere:

#a^3 = 4/3pir^3#

We need to manipulate #4/3pir^3# to get the required result.

Since #4/3pir^3# can be expressed #(4pir^3)/3# This is just a fraction like any other, so we can multiply numerator and denominator by #9#

This then gives:

#(36pir^3)/27#

Now we have:

#a^3 = (36pir^3)/27#

Taking cube roots:

#root(3)(a^3) = root(3)((36pir^3)/27) => root(3)(a^3) = (root(3)(36pir^3))/(root(3)(27))#

Extracting and evaluating any cubes:

#a = (rroot(3)(36pi))/3#

Which can be expressed:

#a = (root(3)(36pi))/3r#