The triangle can be divided into #3# congruent triangle by drawing lines from the center to the vertices. (Sorry if my diagram does not appear to have congruent sub-triangles; they really are congruent).
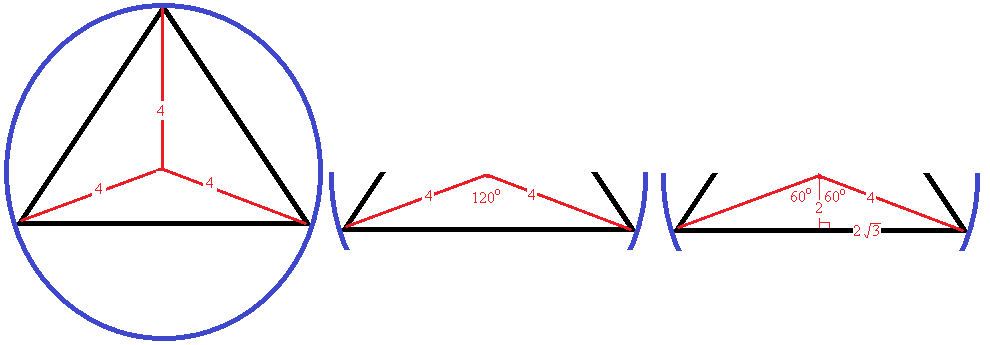
Each of these #3# sub-triangles can be divided into #2# sub-sub-triangles with a #60^circ#, a right angle, and a hypotenuse of length #4#
The #60^circ# right-angled triangle is one of the standard triangles
and given a hypotenuse of #4#
the other two sides will have lengths #2# and #2sqrt(3)# as indicated above.
The Area of each of these sub-sub-triangles will be
#color(white)("XXX")A_"sst"=(2xx2sqrt(3))/2 = 2sqrt(3)#
Since the original triangle is composed of #6# such sub-sub-triangles
the area of the original triangle must be
#color(white)("XXX")6xx2sqrt(3)=12sqrt(3)#

