Question

In which hydride is the H-X-H bond angle the smallest?

In which hydride is the H-X-H bond angle the smallest?

A.`BH_3`
B. `CH_4`
C. `C_2H_6`
D.`NH_3`

Answer 1

Well, it's not entirely obvious. It is either `"C"_2"H"_6` or `"NH"_3`, but we would need more accurate data to discriminate, and we could not have figured this out from purely qualitative arguments.

CCCBDB gives:

`color(blue)(/_"HCH" = 108^@)`
http://cccbdb.nist.gov/exp2x.asp?casno=74840

`color(blue)(/_"HNH" = 106.67^@)`
http://cccbdb.nist.gov/exp2x.asp?casno=7664417

And so, as it turns out, the lone-pair-bonding-pair repulsion in `"NH"_3` dominated the bonding-pair-bonding-pair repulsion in `"C"_2"H"_6`.

---

Well, if you notice, the first three are all ideal geometries. If you don't notice that, consider their Lewis structures and how they have zero lone pairs of electrons on the central atom:

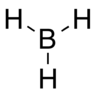
`120^@` angle, trigonal planar

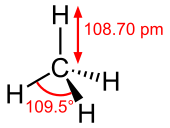
`109.5^@` angle, tetrahedral

---

Here's where we run into some trouble.

`109.5^@` predicted, and we get `111.17^@` for the `"H"-"C"-"C"` bond angle, which got bigger.

This is likely due to the `"C"-"H"//"C"-"H"` bonding-pair-bonding-pair charge repulsions from each half (each `"CH"_3`) of the molecule.

Using conceptual math, an increase of `111.17 - 109.50 = 1.67^@` in one direction is a decrease of roughly `1.67^@` in the other, depending on how much the `"C"-"H"` bonding pairs repel each other within the same `"CH"_3` fragment.

This predicts roughly `107-108^@` for the `"H"-"C"-"H"` bond angle...

---

On the other hand, `"NH"_3` has a lone pair of electrons, which compresses the three `"N"-"H"` bonds down due to charge repulsions between the lone pair and the bonding pairs:

This image suggests a bond angle of `106.7^@`, which apparently is the smallest here. It's close, but... probably `"NH"_3`.