Resultant Forces using simultaneous equations?
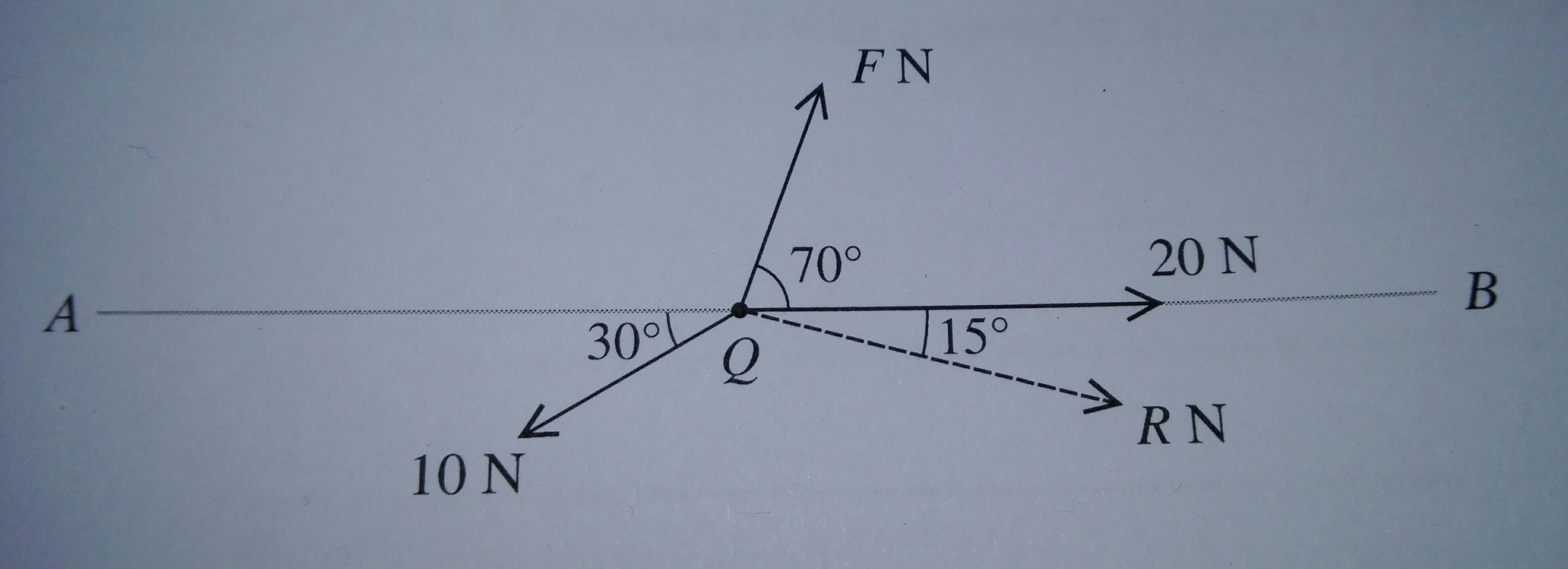
A small bead #Q# can move freely along a smooth horizontal straight wire #AB# of length #3m# . Three horizontal forces of magnitudes #FN# , #10N# and #20N# act on the bead in the directions shown in the diagram. The magnitude of the resultant of the three forces is #RN# in the direction shown in the diagram.
(i) Find the values of #F# and #R# . #[5]#
I have gone over this question three times after looking at the mark scheme but still can't seem to get it right. Would be really helpful if someone can show me all the necessary steps to get the correct answer.
#F = 1.90N# and #R = 12.4N# solved using simultaneous equations.

A small bead
(i) Find the values of
I have gone over this question three times after looking at the mark scheme but still can't seem to get it right. Would be really helpful if someone can show me all the necessary steps to get the correct answer.
1 Answer
Resolve given three horizontal forces of magnitudes
We know that magnitude of resultant must be equal to the sum of magnitudes of components of three forces in its direction. Also that the sum of perpendicular components of three forces must be zero.
Angle between forces
Angle between force
If direction along
#R=20cos15+Fcos85-10cos45# .....(1)
#0=20sin15+Fsin85-10sin45# .....(2)
From (2)
#0=5.18+Fsin85-7.07#
#=>F=1.89/sin85=1.90# N
Inserting this value in (1)
#R=20cos15+1.90cos85-10cos45#
#=>R=12.41# N
