The correct option among the following is?
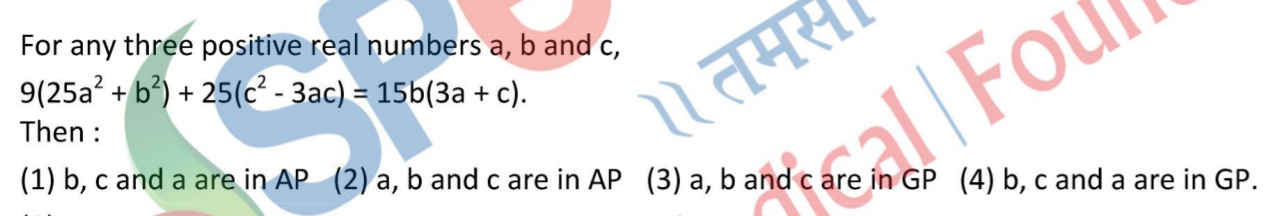
1 Answer
Feb 27, 2018
Answer is (1).
Explanation:
We can write
or
or
This is of type
and we can write it as
or
As sum of three squares is
and hence
Hence our
and
Hence answer is (1).
