The curve #y = 2x^3 - 6x^2 - 2x + 1# has two tangents parallel to the line #2x + y = 12#. Find their equations?

Question

#My solution is

y^1 = 6x^2 - 12x - 2
Mt = -2x + 12

m = -2

-2 = 6x^2 - 12x + 2
0 = 6x - 12
(12/6) = x = 2

y = 2(2)^3 - 6(2)^2 - 2(2) + 1
= 11

The two equation solutions are
2x + y -1 = 0, 2x + y + 7 = 0#

6725 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-01-31T21:49:37

The two tangent equations are:

# y = -2x + 1 #
# y = -2x -7 #

The gradient of the tangent to a curve at any particular point is given by the derivative of the curve at that point.

so If # y = 2x^3-6x^2-2x+1 # then differentiating wrt gives us:

# dy/dx = 6x^2-12x-2 #

If we examine the given line and rewrite in the form #y=mx+c#, we get:

# 2x+y=12 => y = -2x+12#

So the slope of this line is #-2#, and so we set #dy/dx=-2# and solve for #x#

# 6x^2-12x-2 = -2 #
# :. 6x^2-12x = 0 #
# :. 6x(x-2) = 0 #
# :. x = 0,2 #

We now have the #x#-coordinate of the two points, so we must find the corresponding #y#-coordinate and the tangent equation using:

#y-y_1 = m(x-x_1)#:

Consider the case #x=0#:

# x=0 => y = 0-0-0+1 = 1 #

# :. y-1 = (-2)(x-0) #
# :. y-1 = -2x #
# :. \ \ \ \ \ \ \y = -2x + 1 #

Consider the case #x=2#:

# x=2 => y = 16-24-4+1 = -11#

# :. y-(-11) = (-2)(x-2) #
# :. \ \ \ \ \ \ \ \ y+11 = -2x +4#
# :. \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ y = -2x -7 #

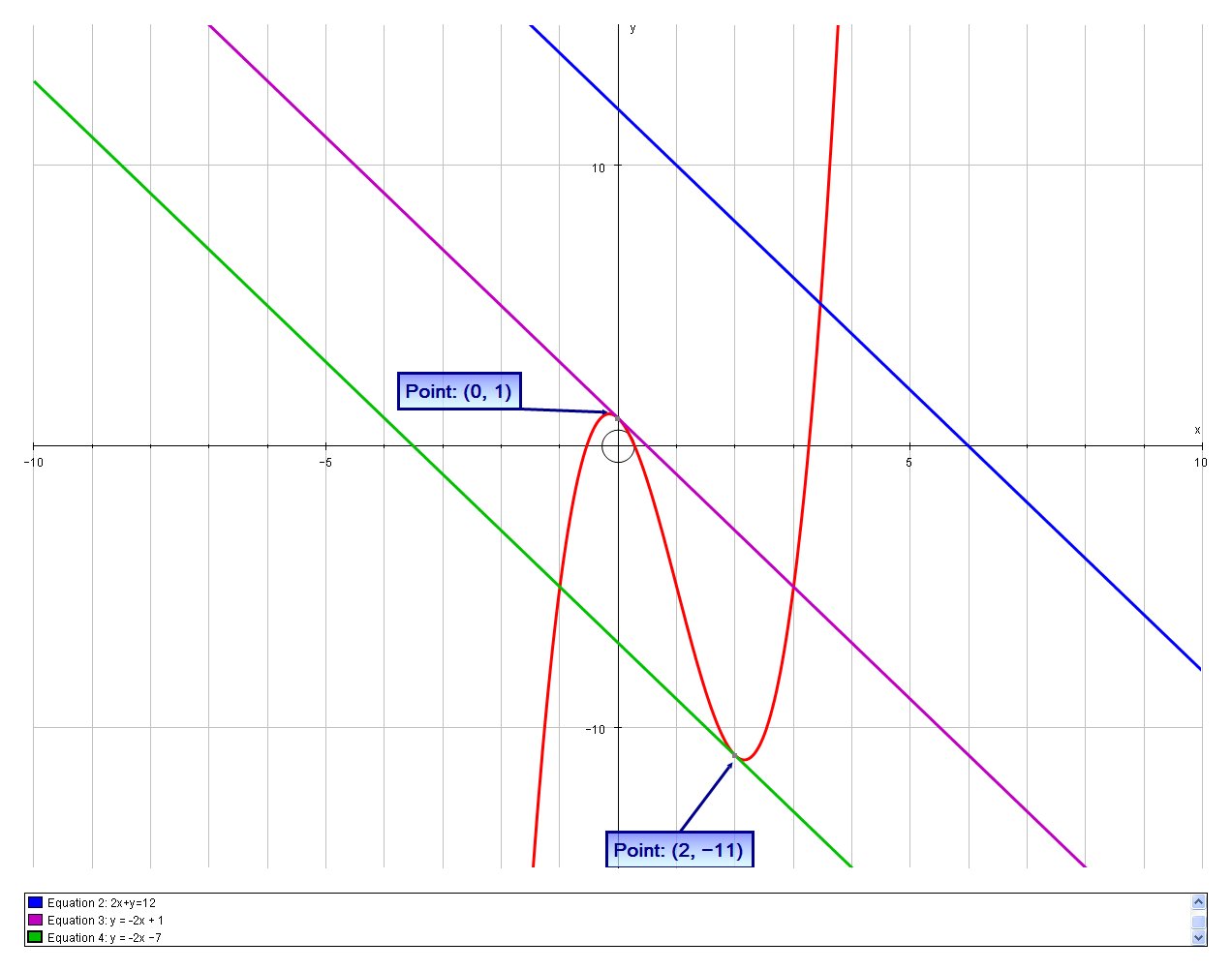
We can confirm this solution is correct graphically:

enter image source here