Three identical point charges, each of mass m =0 .100kg and charge q hang from three strings. If the lengths of the left and right strings are L = 30 cm and the angle with vertical is θ = 45 .0◦, What is the value of charge q?

enter image source here

1 Answer
P dilip_k
Feb 17, 2017

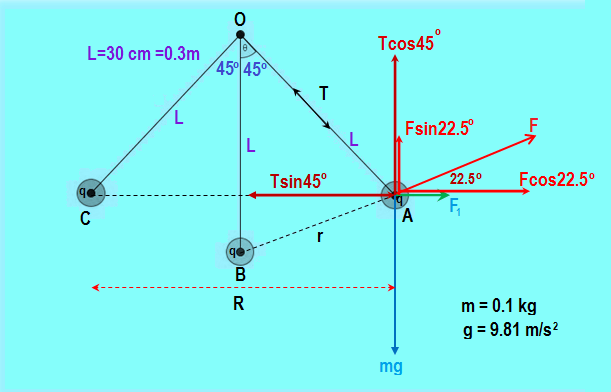
drawn

The situation as described in the problem is shown in above figure.
Let the charges on each point charges (A,B,C) be #qC#

In #Delta OAB,/_OAB=1/2(180-45)=67.5^@#
So #/_CAB=67.5-45=22.5^@#

#/_AOC=90^@#

So #AC^2=OA^2+OC^2=2L^2#

#=>R^2=2L^2#

For #Delta OAB,#

#AB^2=OA^2+OB^2-2OA*OBcos45^@#

#=>r^2=L^2+L^2-2L^2xx1/sqrt2=L^2(2-sqrt2)#

Now forces acting on A

Electrical repulsive force of B on A

#F=k_eq^2/r^2#

Electrical repulsive force of C on A

#F_1=k_eq^2/R^2#

where #k_e="Coulomb's const"=9xx10^9Nm^2C^-2#

#F/F_1=R^2/r^2=sqrt2/(2-sqrt2)=(sqrt2(2+sqrt2))/((2+sqrt2)(2-sqrt2))#
#=(2sqrt2+2)/2=sqrt2+1#

And #T="Tension on string"#

Considering equilibrium of forces acting on A we can write

For vertical forces on A

#Tcos45+Fsin22.5=mg#

#=>T/sqrt2=mg-Fsin22.5........[1]#

For horizontal forces on A

#Tsin45=Fcos22.5+F_1#

#=>T/sqrt2=Fcos22.5+F_1........[2]#

Comparing [1] an [2] we get

#Fcos22.5+F_1=mg-Fsin22.5#

#=>Fcos22.5+Fsin22.5+F_1=mg#

#=>F(cos22.5+sin22.5)+F_1=mg#

#=>F(sqrt(cos^2 22.5+sin^2 22.5+2sin22.5xxcos22.5))+F_1=mg#

#=>F(sqrt(1+sin45))+F_1=mg#

#=>F(sqrt(1+1/sqrt2))+F_1=mg#

#=>F(sqrt((2+sqrt2)/sqrt2))+F_1=mg#

#=>F_1xx(sqrt2+1)(sqrt((2+sqrt2)/sqrt2))+F_1=mg#

#=>F_1[(sqrt2+1)(sqrt((2+sqrt2)/sqrt2))+1]=0.1xx9.81#

#=>F_1xx6.47=0.1xx9.81#

#=>k_eq^2/R^2=(0.1xx9.81)/6.47~~0.152#

#=>q=Rxxsqrt(0.152/k_e)#

#=>q=sqrt2Lxxsqrt(0.152/k_e)#

#=>q=sqrt2xx0.3xxsqrt(0.152/(9xx10^9))C=1.74muC#

Related questions
Impact of this question
13171 views around the world
You can reuse this answer
Creative Commons License