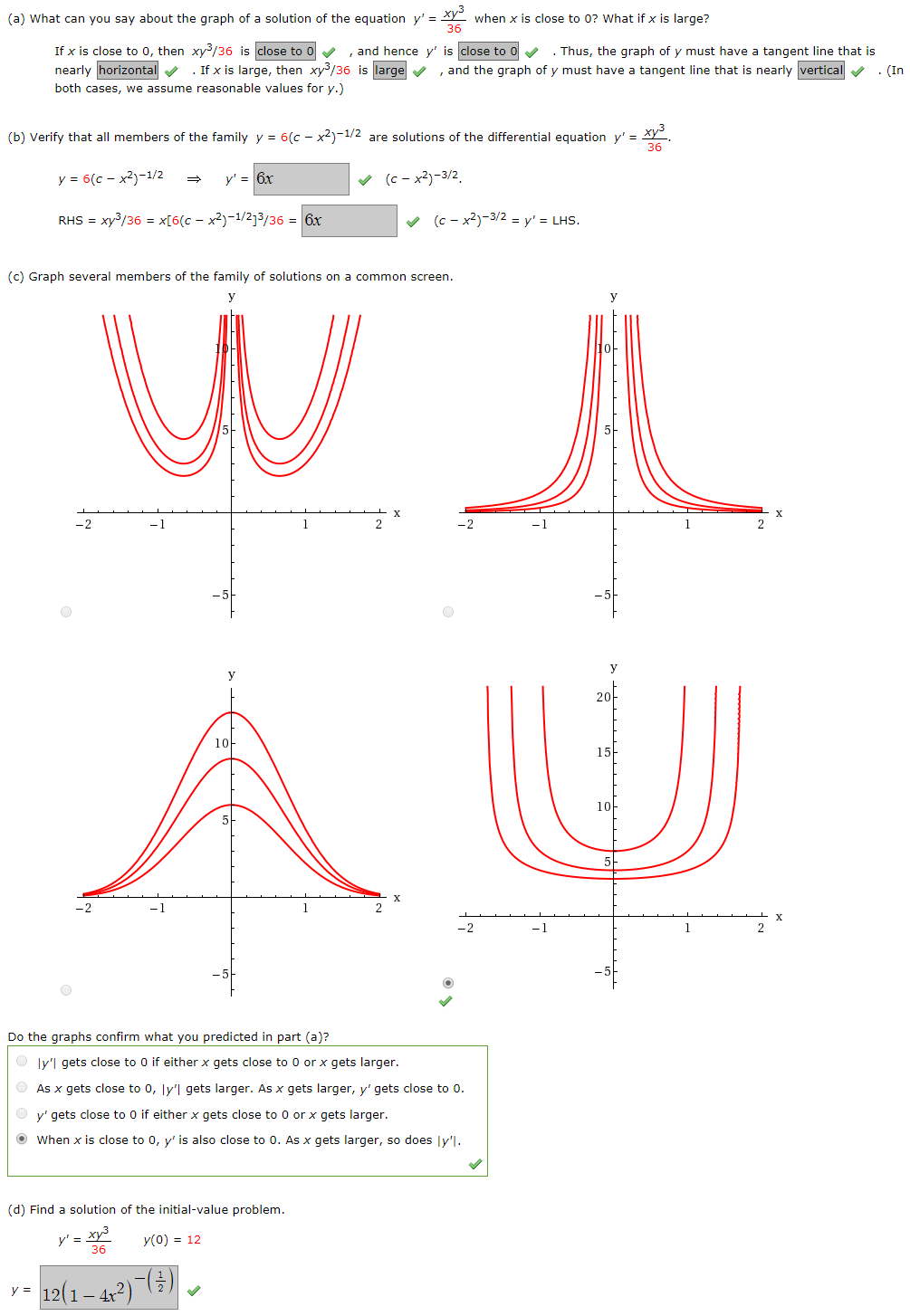
What can you say about the graph of a solution of the equation y' = #(xy^3)/36# when x is close to 0?
(a) What can you say about the graph of a solution of the equation y' = #(xy^3)/36# when x is close to 0? What if x is large?
If x is close to 0, then y' = #(xy^3)/36# is (choices are: large, close to 0, and close to 36) , and hence y' is (choices are: large, close to 0, and close to 36) Thus, the graph of y must have a tangent line that is nearly (choices are: horizontal, vertical). If x is large, then #(xy^3)/36# is (choices are: large, close to 0, and close to 36), and the graph of y must have a tangent line that is nearly (choices are: horizontal, vertical). (In both cases, we assume reasonable values for y.)
(b) Verify that all members of the family y = 6#(c - x^2)^(-1/2)# are solutions of the differential equation y' = #(xy^3)/36# .
y = 6#(c - x^2)^(-1/2)# => y' = (blank) #(c - x^2)^(-3/2)# .
RHS = #(xy^3)/36# = #(x[6(c - x^2)^(-1/2))]/36# = (blank) #(c - x^2)^(-3/2)# = y' = LHS.
(c) Graph several members of the family of solutions on a common screen.
 or
or 
Do the graphs confirm what you predicted in part (a)?
(A) |y'| gets close to 0 if either x gets close to 0 or x gets larger.
(B) As x gets close to 0, |y'| gets larger. As x gets larger, y' gets close to 0.
(C) y' gets close to 0 if either x gets close to 0 or x gets larger.
(D) When x is close to 0, y' is also close to 0. As x gets larger, so does |y'|.
(d) Find a solution of the initial-value problem.
y' = #(xy^3)/36# , y(0) = 12
y = ???
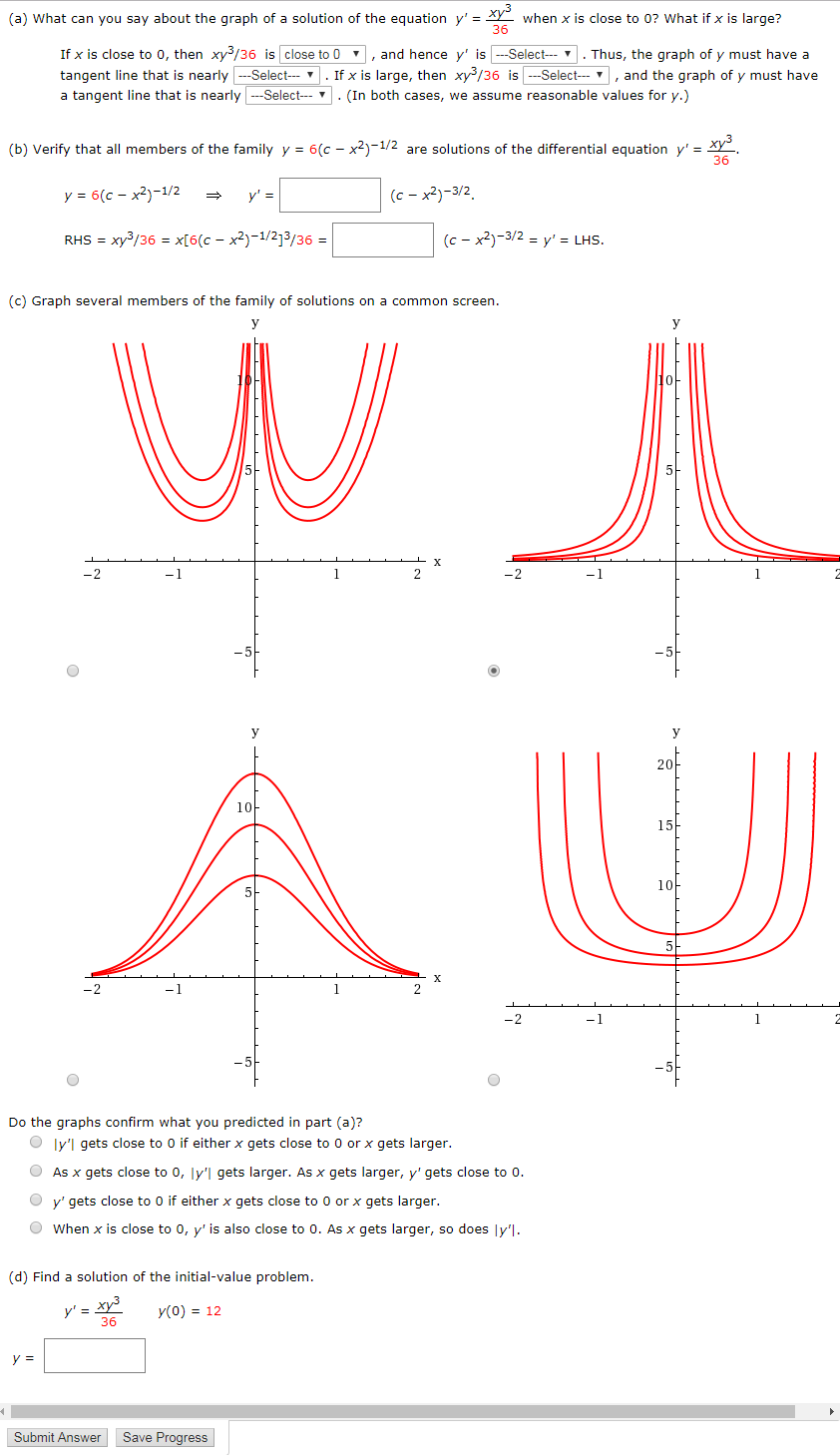
How can I do parts a, b, and c? I think I got the graphing part of c just fine, but I'm not sure. How can I do the rest?
(a) What can you say about the graph of a solution of the equation y' =
If x is close to 0, then y' =
(b) Verify that all members of the family y = 6
y = 6
RHS =
(c) Graph several members of the family of solutions on a common screen.
 or
or 
Do the graphs confirm what you predicted in part (a)?
(A) |y'| gets close to 0 if either x gets close to 0 or x gets larger.
(B) As x gets close to 0, |y'| gets larger. As x gets larger, y' gets close to 0.
(C) y' gets close to 0 if either x gets close to 0 or x gets larger.
(D) When x is close to 0, y' is also close to 0. As x gets larger, so does |y'|.
(d) Find a solution of the initial-value problem.
y' =
y = ???

How can I do parts a, b, and c? I think I got the graphing part of c just fine, but I'm not sure. How can I do the rest?
1 Answer
(a) Close to 0 / Close to 0 / Horizontal / Large / Vertical
(b) Put 6x for both boxes.
(c) 
(d) y =
Explanation: