What is the complex conjugate for the number #7-3i#?
1 Answer
Dec 20, 2014
the complex conjugate is:
To find your complex conjugate you simply change sign of the imaginary part (the one with
So the general complex number:
Graphically:
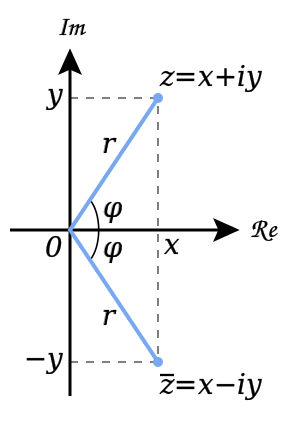
(Source: Wikipedia)
An interesting thing about complex conjugate pairs is that if you multiply them you get a pure real number (you lost the
(Remembering that:

