Question

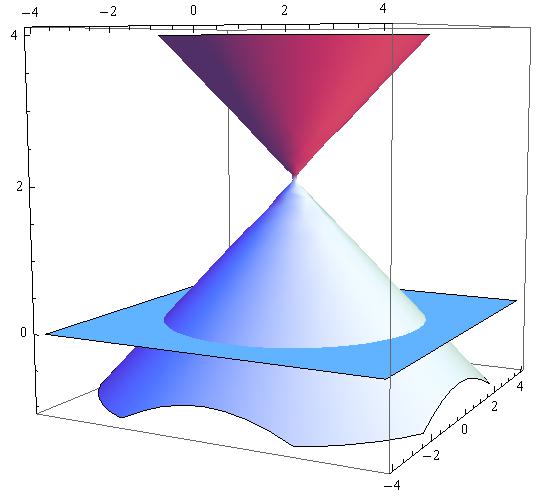
What is the equation of the right circular cone when the straight line `2y+3z=6, x=0` revolve about the `z` axis?

Answer 1

See below.

Explanation:

Given the vertex point

`p_0=(x_0,y_0,z_0) = (0,0,2)` and `p = (x,y,z)`

the support circle in the `x xx y` plane

`x^2+y^2 = r^2`

with `r = 3`

The generatrix line is given as

`(x-x_0)/l=(y-y_0)/m=(z-z_0)/n`

so it's intersection with the `x xx y` plane is given by

`(x_0-z_0(l/n),y_0-z_0(m/n), 0)`

so this point will lie on the given circle

`(x_0-z_0(l/n))^2+(y_0-z_0(m/n))^2=r^2`. Here we have

`(2(l/n))^2+(2(m/n))^2=3^2`

but `{(l = x/k),(m=y/k),(n=(z-2)/k):}` substituting

`(2(x/(z-2)))^2+(2(y/(z-2)))^2 = 3^2`

and finally

`x^2+y^2-(3/2)^2(z-2)^2=0`

Attached a plot.