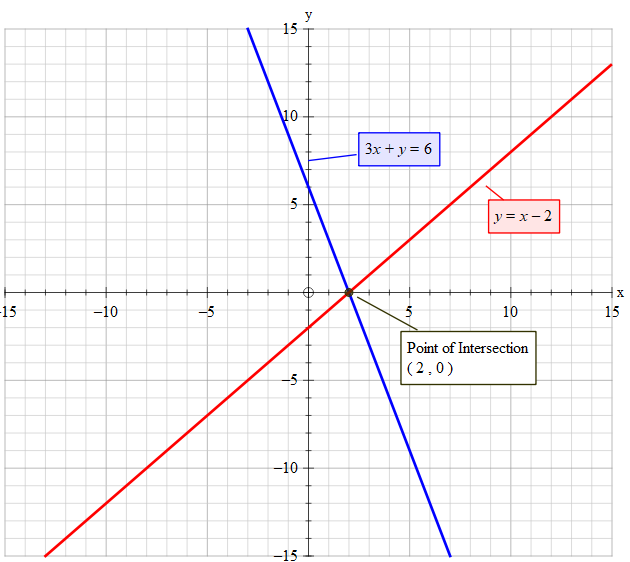
Given:
#3x+y=6" ".....................Equation(1)#
#y=x-2" "......................Equation(2)#
#color(blue)("Determine the value of "x)#
Using #Eqn(2)# substitute for #color(red)(y)# in #Eqn(1)# giving:
#color(green)(3x+color(red)(y)color(white)("d")=color(white)("d")6 color(white)("dddd") ->color(white)("dddd")3x+(color(red)(x-2))color(white)("d")=color(white)("d")6)#
#color(green)(color(white)("ddddddddddd.d")->color(white)("dddddd") 4xcolor(white)("ddd")-2color(white)("ddd")=color(white)("d")6)#
Add #color(red)(2)# to both sides
#color(green)(4x-2color(white)("d")=color(white)("d")6 color(white)("dddd") ->color(white)("dddd")4xcolor(white)("d")-2color(red)(+2)color(white)("dd")=color(white)("d")6color(red)(+2))#
#color(green)(color(white)("ddddddddddddd.d")->color(white)("dddd") 4xcolor(white)("d")+color(white)("d")0color(white)("dddd")=color(white)("dd")8#
Divide both sides by #color(red)(4)#
#color(green)(4xcolor(white)("d")=color(white)("d")8color(white)("dddddd.d")->color(white)("dddd") 4/color(red)(4)xcolor(white)("d")=color(white)("d")8/color(red)(4) )#
# color(green)(color(white)("ddddddddddddd.d")->color(white)("dddddd") xcolor(white)("d")=color(white)("d")2 )#
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
#color(blue)("Determine the value of "y)#
Substitute for #color(red)(x=2) # in #Eqn(1)#
#color(green)(ycolor(white)("d")=color(white)("d")color(red)(x)-2 color(white)("dddd")->color(white)("dddd")ycolor(white)("d")=color(white)("d")color(red)(2)-2)#
#color(green)(color(white)("dddddddddddddd")->color(white)("dddd")ycolor(white)("d")=color(white)("d")0)#
#color(magenta)("So the point common to both plots is "(x,y)->(2,0))#