What should I put for using the integral test to determine whether the series is convergent or divergent?
DIVERGES and DIVERGENT doesn't work.
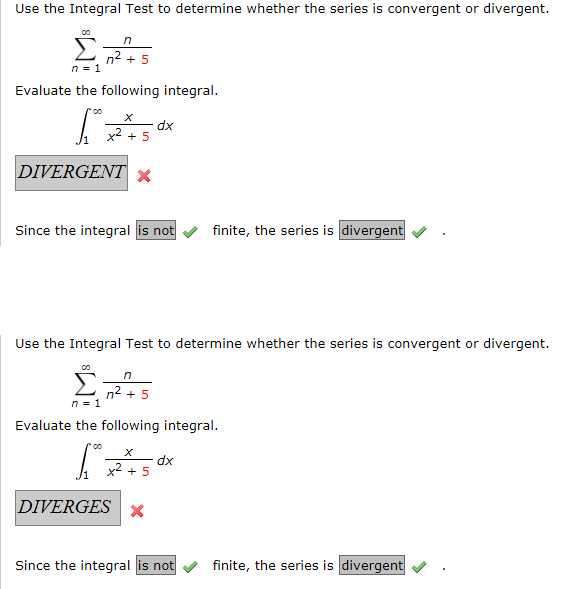
~~~~~~~~~~~~~~~~~
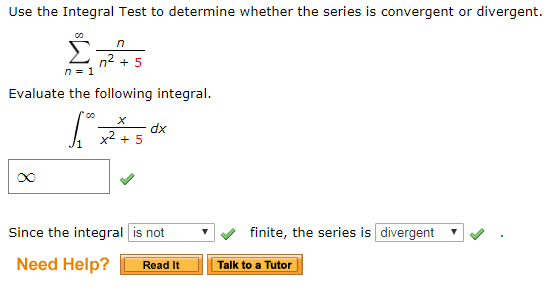
DIVERGES and DIVERGENT doesn't work.

~~~~~~~~~~~~~~~~~

1 Answer
Jul 2, 2017
The series:
is divergent.
Explanation:
Based on the integral test, the convergence of the series:
is equivalent to the convergence of the improper integral:
as the function
Evaluate the indefinite integral:
then:
So the series is divergent.

