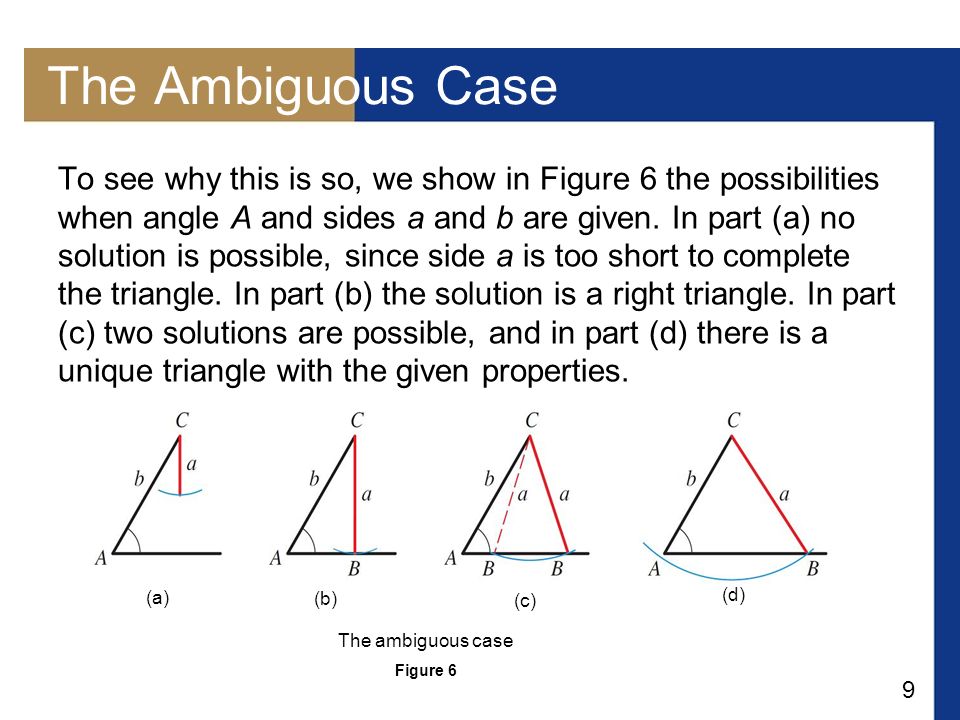
The Ambiguous Case

Key Questions

• How do you know when to use the ambiguous case when finding possible lengths of triangles?

  Answer:

  As listed below.

  Explanation:

  For those of you who need a reminder, the ambiguous case occurs when one uses the law of sines to determine missing measures of a triangle when given two sides and an angle opposite one of those angles (SSA). ... If angle A is acute, and a = h, one possible triangle exists

  1. If angle A is acute, and a < h, no such triangle exists.

  2. If angle A is acute, and a = h, one possible triangle exists.

  3. If angle A is acute, and a > b, one possible triangle exists.

  4. If angle A is acute, and h < a < b, two possible triangles exist.

  5. If angle A is obtuse, and a < b or a = b, no such triangle exists.

  6. If angle A is obtuse, and a > b, one such triangle exists.

  

  sankarankalyanam · 1 · Mar 29 2018
• If you have a SSA angle case with two possible solutions, how can you check both solutions to make sure they are correct?

  Three numbers (#a,b,c#) can be the lenght of three sides of a triangle if and only if each of them is greater then the difference of the other two, and less of the sum of the other two.

  I.E: (if #a>b>c#)

  #a>b-c#,
  #b>a-c#,
  #c>a-b#;

  and:

  #a<b+c#,
  #b<a+c#,
  #c<a+b#.

  Massimiliano · 1 · Feb 14 2015
• How do you find the second triangle in the ambiguous case?

  Answer:

  As listed below.

  Explanation:

  If the sum is over 180°, then the second angle is not valid. First we know that this triangle is a candidate for the ambiguous case since we are given two sides and an angle not in between them. We need to find the measure of angle B using the Law of Sines: If their sum is less than 180°, we know a triangle can exist.

  (http://www.softschools.com/math/calculus/the_ambiguous_case_of_the_law_of_sines/)

  To determine if there is a 2nd valid angle:

  1. See if you are given two sides and the angle not in between (SSA). This is the situation that may have 2 possible answers.

  2. Find the value of the unknown angle.

  3. Once you find the value of your angle, subtract it from 180° to find the possible second angle.

  4. Add the new angle to the original angle. If their sum is less than 180°, you have two valid answers. If the sum is over 180°, then the second angle is not valid.

  5. If already one obtuse angle given, it can not have a second set of values.

  sankarankalyanam · 1 · Mar 26 2018
• What is ambiguous case of triangle?

  To solve a triangle it is necessary to know at least three elements with an only exception: if these three elements are the three angles.

  In fact if two triangles have identical the three angles, they are similar.

  Massimiliano · 1 · Jan 30 2015

• How do you know when to use the ambiguous case when finding possible lengths of triangles?
• How do you find the second triangle in the ambiguous case?
• What is ambiguous case of triangle?
• How do you find possible triangles given two sides and an angle (SSA)?
• How do you use the Law of Cosines and Sines in various ambiguous cases?
• How do you find #angle B# if in triangle ABC, #angleA=41^@#, #AC=23#, #CB=12#?
• How do you know how many triangles are created given A = 61°, a = 23, b = 24?
• How do you determine the number of possible triangles and find the measure of the three angles given #a=8, b=10, mangleA=20#?
• How do you determine the number of possible triangles and find the measure of the three angles given #a=5, b=10, mangleA=30#?
• How do you determine the number of possible triangles and find the measure of the three angles given #b=12, c=10, mangleB=49#?
• How do you determine the number of possible triangles and find the measure of the three angles given #a=6, c=10, mangleA=45#?
• How do you determine the number of possible triangles and find the measure of the three angles given #c=18, b=10, mangleC=120#?
• How do you determine the number of possible triangles and find the measure of the three angles given #a=9, c=10, mangleC=150#?
• How do you determine the number of possible triangles and find the measure of the three angles given #AB=14, BC=21, mangleC=75#?
• How do you determine the number of possible triangles and find the measure of the three angles given #DE=24, EF=18, mangleD=15#?
• How do you determine the number of possible triangles and find the measure of the three angles given #PQ=12, PR=15, mangleR=100#?
• How do you determine the number of possible triangles and find the measure of the three angles given #BC=12, AC=12sqrt2, mangleB=135#?
• How do you determine the number of possible triangles and find the measure of the three angles given #RS=3sqrt3, ST=3, mangleT=60#?
• How do you determine the number of possible triangles and find the measure of the three angles given #a=8, b=10, mangleA=45#?
• Can someone help me solve this problem?