How do you integrate #int 1/sqrt(e^(2x)+12e^x-45)dx# using trigonometric substitution?
2 Answers
where
Explanation:
Completing the square at the denominator gives
To make use of the identity
substitute
nice.. ...and the mighty Pythagorean right triangle supports this....
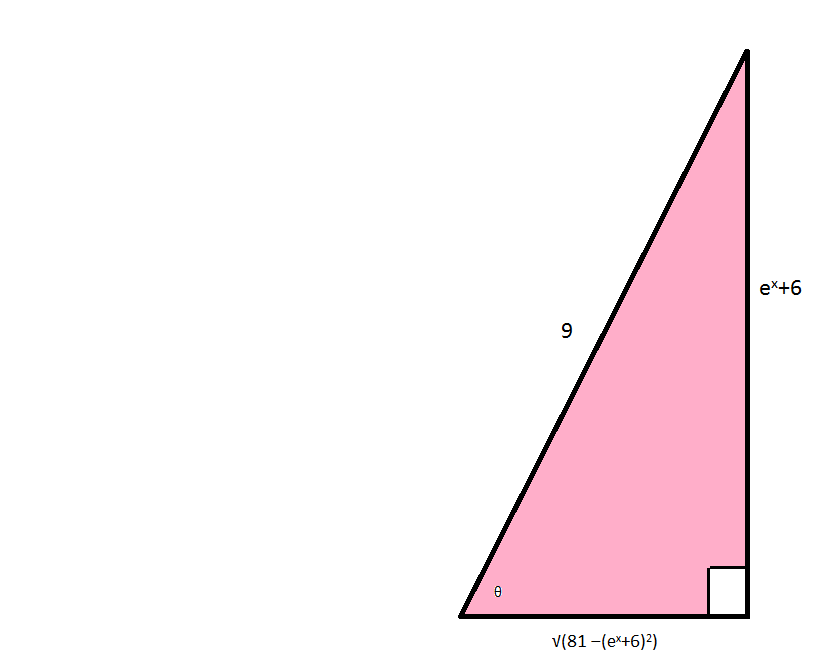
From this tiny little triangle you can glean TONS of valuable information and insight. Simple geometries can typically yield a beautiful perspective of things. On your own and using this triangle as your reference, find:
No need to memorize formulas......that is WAY too much work and expended effort!!
Explanation:
Ok it work my bad


