What is the limit of #(1 + 2/x)^x# as x approaches infinity?
2 Answers
Explanation:
Use
# = lim_(xrarroo)((1+1/(x/2))^(x/2))^2#
# = (lim_(xrarroo)(1+1/(x/2))^(x/2))^2#
Now we have the form above with
# = e^2#
Jim, awesome ..
What this limit really represents is essentially the horizontal asymptote y =
Explanation:
Here are a couple of TI screenshots showing the graph and the decimal expansion for
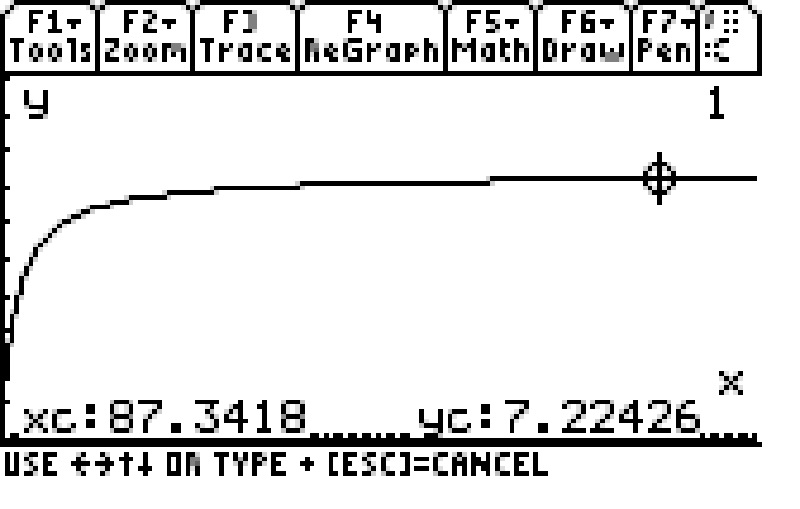
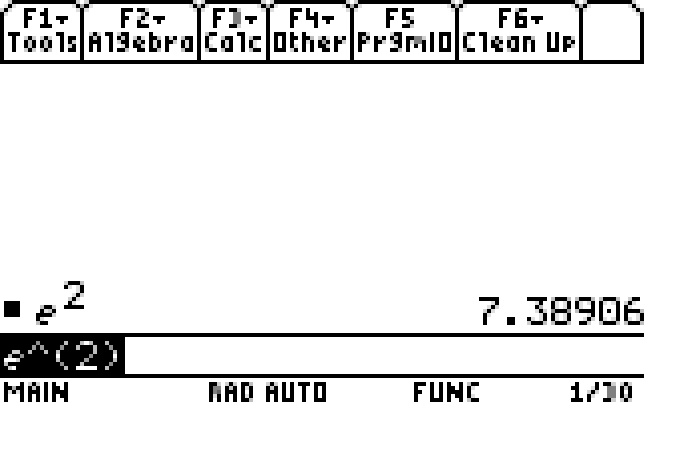
If we went even further out to the right and then asked some random guy on the street if they are looking at a straight line, they would say "yes!".

But in fact you are looking at the curve endowed with concavity, not a straight line. The curve is asymptotically approaching the value of


