(A)
Carbonic acid is a weak diprotic acid with 2 ionisations:
H_2CO_3rightleftharpoonsHCO_3^(-)+H^+" "K_(a1)=4.27xx10^(-7)"mol/l"
HCO_3^(-)rightleftharpoonsCO_3^(2-)+H^(+)" "K_(a2)=4.68xx10^(-11)"mol/l"
In the flask we have 20.00"ml" of 0.10"M" sodium carbonate. As the acid is run in from the burette the following reaction occurs as the carbonate ion becomes protonated:
CO_3^(2-)+H^+rarrHCO_3^(-)
The initial number of moles of CO_3^(2-) is given by:
nCO_3^(2-)=20.00xx0.10/1000=2.00xx10^(-3)
At 0.05"ml" before the end-point we have added 19.05"ml" of 0.10"M"color(white)xHCl"_((aq)).
The number of moles of HCl added is given by:
nHCl=19.05xx0.10/1000=1.905xx10^(-3)
This will be equal to the number of moles of HCO_3^- formed.
So the number of moles of CO_3^(2-) remaining is given by:
nCO_3^(2-)=(2.00xx10^(-3)-1.905xx10^(-3))=0.095xx10^(-3)
To get the number of moles of H^+ released we can use an ICE table based on "mol/l"
color(white)(xxx)HCO_3^(-)color(white)(xxxxx)rightleftharpoonscolor(white)(xxxx)CO_3^(2-)color(white)(xxxxx)+color(white)(xxxxx)H^+
color(red)"I"color(white)(xxx)1.905xx10^(-3)color(white)(xxxxxx)0.095xx10^(-3)color(white)(xxxxxxxx)0
color(red)"C"color(white)(xxxx)-xcolor(white)(xxxxxxxxxxxxxx)+xcolor(white)(xxxxxxxxx)+x
color(red)"E"color(white)(xxx)(1.905xx10^(-3))-xcolor(white)(xxx)(0.0905xx10^(-3))+xcolor(white)(xx)x
Since x is much smaller than nHCO_3^- and nCO_3^(2-) we can say that at equilibrium:
:.nHCO_3^(-)=(1.905xx10^(-3))-xrArr(1.905xx10^(-3))
and:
nCO_3^(2-)=(0.095xx10^(-3))+xrArr(0.095xx10^(-3))
I will make this approximation in the rest of the calculations.
The expression for K_(a2) is:
K_(a2)=([CO_3^(2-)][H^+])/[[HCO_3^-]]
Since the total volume is common to all species we can write:
:.[H^+]=4.68xx10^(-11)xx(1.905xxcancel10^(-3))/(0.095xxcancel(10^(-3))
:.[H^(+)]=9.38xx10^(-10)
:.pH=-log(9.38xx10^(-10))
color(red)(pH=9.02
This represents the pH just before the 1st end-point.
(B)
At the end-point the 1st protonation is complete:
CO_3^(2-)+H^(+)rarrHCO_3^-
So we now have 40.00"ml" of a solution containing 2.00xx10^(-3) moles of HCO_3^- ions.
As we continue to add acid the 2nd protonation starts to happen:
HCO_3^(-)+H^+rarrH_2CO_3
After the end point at 20.00"ml" we add 0.05"ml" of 0.1"M"color(white)(x)"HCl":
The number of moles of H^+ added is given by:
nH^+=0.05xx0.10/1000=5.00xx10^(-6)
From the equation of the reaction for this stage of the titration we can say that:
nH_2CO_3 formed =5.00xx10^(-6)
So the number of moles of HCO_3^- remaining is given by:
nHCO_3^(-)=(2.00xx10^(-3))-(5.00xx10^(-6))=0.001995
K_(a1)=([HCO_3^-][H^+])/([H_2CO_3])
:.[H^+]=K_(a1)xx([H_2CO_3])/([HCO_3^-])
:.[H^+]=4.27xx10^(-7)xx(5.00xx10^(-6))/(0.001995)=1.0675xx10^(-9)
:.pH=-log(1.0675xx10^(-9))
color(red)(pH=8.97)
Phenolphthalein is an indicator that changes colour over the pH range 8.3 - 10 so is a suitable indicator for this end-point.
(C)
Now the titration continues to 0.05"ml" short of the 2nd end-point which occurs at 40.00"ml" of "HCl" added.
Since the 1st end-point we have added 19.05"ml" of 0.10"M"color(white)(x)" HCl".
:.nH^+ added =19.05xx0.10/1000=1.905xx10^(-3)
:.nH_2CO_3 formed =1.905xx10^(-3)
:.nHCO_3^- remaining = (2.00xx10^(-3))-(1.905xx10^(-3))=0.095xx10^(-3)
K_(a1)=([H^+][HCO_3^-])/([H_2CO_3])
:.[H^+]=K_(a1)xx[[H_2CO_3]]/[[HCO_3^(-)]]
:.[H^+]=4.27xx10^(-7)xx(1.905xxcancel(10^(-3)))/(0.095xxcancel(10^(-3)
[H^+]=8.561xx10^(-6)
pH=-log(8.561xx10^(-6))
color(red)(pH=5.06)
(D)
When we get to the end point this reaction has reached completion:
Na_2CO_(3(aq))+2HCl_((aq))rarrH_2CO_(3(aq))+2NaCl_((aq))
So we have 60"ml" of a solution that contains 2xx10^(-3) moles of H_2CO_3.
We can find the pH of this solution. I will ignore the 2nd ionisation because K_(a2) is much smaller than K_(a1).
K_(a1)=([HCO_3^-][H^+])/([H_2CO_3])
Since [H^+]=[HCO_3^-] we can write:
[H^+]^(2)=K_(a1)xx[H_2CO_3]
We can find [H_2CO_3] using c=n/v:
[H_2CO_3]=(2.00xx10^(-3))/(60.00/1000)=0.033"mol/l"
:.[H^+]^2=4.27xx10^(-7)xx0.033
[H^+]^2=1.41xx10^(-8)
:.[H^+]=1.187xx10^(-4)"mol/l"
pH=-log(1.187xx10^(-4))
pH=3.92
Now we add an extra 0.05"ml" of 0.1"M"color(white)(x)" HCl". The extra number of moles of H^+ added is given by:
0.10xx0.05/1000=5.00xx10^(-6)
The number of moles of H^+ which are already there due to the dissociation of H_2CO_3 is given by:
n=cxxv=1.187xx10^(-4)xx60.00/1000=7.122xx10^(-6)
So we add this to the extra H^+ added to get the total moles of H^(+) present:
nH^(+)=(7.122+5)xx10^(-6)
nH^(+)=1.2122xx10^(-5)
The new total volume is 60.05"ml".
:.[H^+]=(1.2122xx10^(-5))/(60.05/1000)=2.00xx10^(-4)"mol/l"
pH=-log(2xx10^(-4))
color(red)(pH=3.7)
So you can see the pH has dropped slightly.
I have ignored any H^+ from the ionisation of water.
The pH curve looks like this:
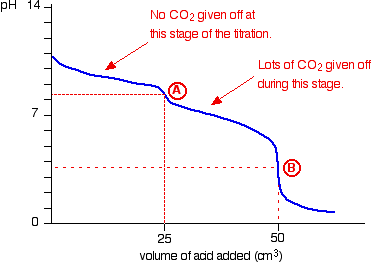
(In our example A is at 20.00ml, B is at 40.00ml)
Methyl Orange is an indicator which changes colour over the range pH 3.1 - 4.4 so is suitable for this end-point.
*


