Question

A triangle has sides A, B, and C. If the angle between sides A and B is `(pi)/8`, the angle between sides B and C is `(5pi)/12`, and the length of B is 1, what is the area of the triangle?

Answer 1

1.23

Explanation:

For sake of easy calculations, the angle measures in degrees would be `angle A = 75^o, angle B=22.5^o and angle C= 82.5^0` as shown in the figure below

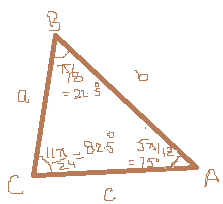
Now using `sin A/a= sin B/b= sin C /c`, it would be

`sin 75 /a= sin 22.5/1= sin 82.5/c`

Thus `a= sin 75/sin 22.5=2.52`; `c= sin 82.5/ sin22.5= 2.59`

Now for using Heron formula for the area of a triangle, `s=(2.52+1+2.59)/2= 6.11/2= 3.05`

(s-a)= 0.53, (s-b)= 2.05, and (s-c)= 0.46

Area of Triangle would be= `sqrt(3.05(0.53)(2.05)(0.46))=1.23`