Question

A triangle has sides A, B, and C. The angle between sides A and B is `(5pi)/12` and the angle between sides B and C is `pi/6`. If side B has a length of 3, what is the area of the triangle?

Answer 1

`2.25`

Explanation:

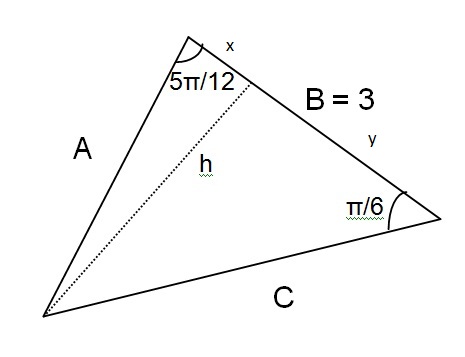
Area `= 1/2*B*h` (see sketch)
`B = x+y`
`tan((5pi)/12) = h/x` and `tan(pi/6) = h/y = h/(B-x)`
`h = xtan((5pi)/12) = (B-x)tan(pi/6)`
`:. xtan((5pi)/12) +xtan(pi/6) = 3tan(pi/6)`
`x = (3tan(pi/6))/(tan((5pi)/12) + tan(pi/6))`
`:. h = tan((5pi)/12)*(3tan(pi/6))/(tan((5pi)/12) + tan(pi/6))`
`~~ (3.73*3*0.577)/(3.73 +0.577)`
`~~6.46/4.31 = 1.5`
Area `=0.5*3*1.5 = 2.25`