Question

If sides A and B of a triangle have lengths of 13 and 2 respectively, and the angle between them is `(pi)/6`, then what is the area of the triangle?

Answer 1

First we solve `pi/6`
`pi=180^circ`

So,

`rarr=180/6=45^circ`

Now we consider the diagram:

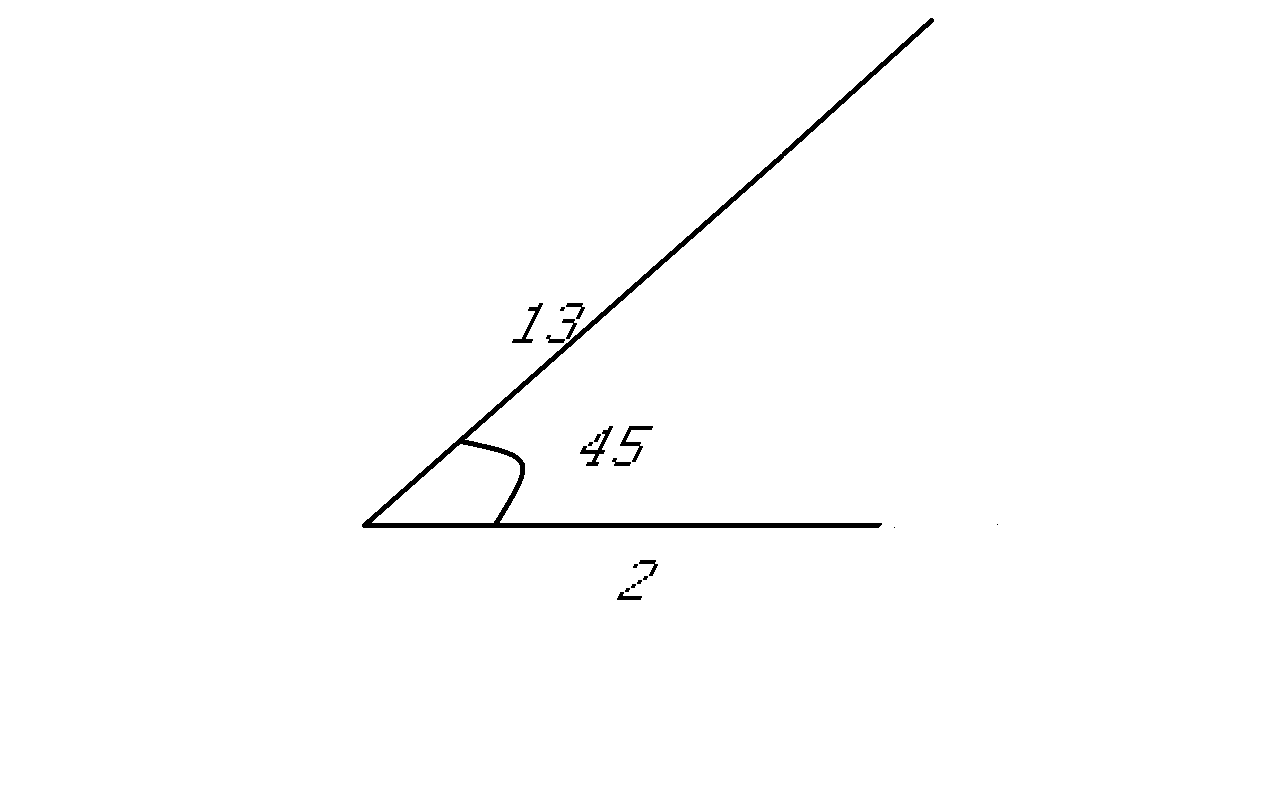
The formula for finding the area of triangle when given two sides of a triangle and the angle between them=`1/2 ab sinC=(ab sinC)/2`

In this case `a=13,b=2,C=45^circ`

`sin C=sin(45^circ)=sqrt2/2`

`rarrArea=((13)(2)(sqrt2/2))/2`

`rarrArea=(26(sqrt2/2))/2`

`rarrArea=((52sqrt2)/2)/2`

`rarrArea=(26sqrt2)/2=13sqrt2`